
Calculate the number of atoms per unit cell of FCC and BCC crystal structure.
Answer
523.2k+ views
4 likes
Hint: Solve the given question using basic knowledge of solid state systems. Solving the question by using diagrams of unit cells would be beneficial.
Complete step by step answer:
This is the structure of a simple or primitive cubic unit cell-
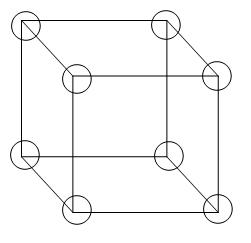
From the diagram we can see that 8 atoms are arranged in all the corners of the cube, therefore, the contribution per unit cell will be 1 atom,
This is the structure of a body-centred unit cell-
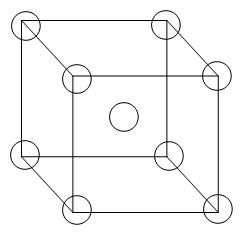
From the diagram we can see that 8 atoms are arranged in all the corners of the cube and there is 1 atom in the centre of the unit cell. Therefore, the contribution per unit cell will be –
= 1 atom (primitive) + 1 atom (body centred)
=2
This is the structure of a face-centred unit cell-
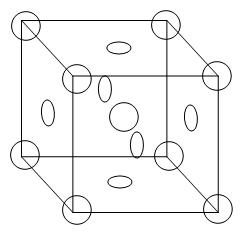
From the diagram we can see that 8 atoms are arranged in all the corners of the cube and there is 1 atom in the centre of the unit cell. In addition to this, there are atoms on each face of the cube. Therefore, the contribution of atoms on the cube =
Therefore, total contribution per unit cell will be –
= 1 atom (primitive) + 1 atom (body centred) + 3 (face centred)
= 4.
Therefore, the answer is –
The number of atoms per unit cell of FCC crystal structure = 4 and,
The number of atoms per unit cell of BCC crystal structure = 2.
Note: Unit cell is the simplest unit of a complete crystal lattice. It is the most uniform unit used for analysis of the structure.
Complete step by step answer:
This is the structure of a simple or primitive cubic unit cell-

From the diagram we can see that 8 atoms are arranged in all the corners of the cube, therefore, the contribution per unit cell will be 1 atom,
This is the structure of a body-centred unit cell-

From the diagram we can see that 8 atoms are arranged in all the corners of the cube and there is 1 atom in the centre of the unit cell. Therefore, the contribution per unit cell will be –
= 1 atom (primitive) + 1 atom (body centred)
=2
This is the structure of a face-centred unit cell-

From the diagram we can see that 8 atoms are arranged in all the corners of the cube and there is 1 atom in the centre of the unit cell. In addition to this, there are atoms on each face of the cube. Therefore, the contribution of atoms on the cube =
Therefore, total contribution per unit cell will be –
= 1 atom (primitive) + 1 atom (body centred) + 3 (face centred)
= 4.
Therefore, the answer is –
The number of atoms per unit cell of FCC crystal structure = 4 and,
The number of atoms per unit cell of BCC crystal structure = 2.
Note: Unit cell is the simplest unit of a complete crystal lattice. It is the most uniform unit used for analysis of the structure.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹34,850 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




