Question

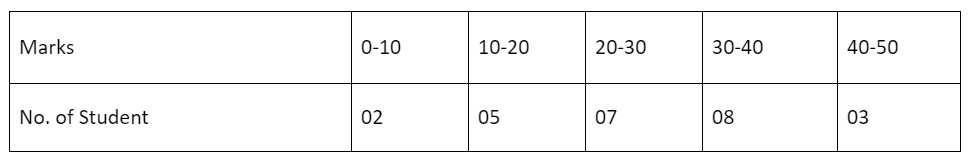
Calculate the range and the coefficient of range with the following data:

Answer 1

Hint: Range is the difference between the highest and the lowest value of frequency for a given frequency distribution. the coefficient of range on the other hand is the ratio of difference between the highest and lowest value of frequency to the sum of highest and lowest value of frequency.

Complete step by step solution:
Now, in this question a frequency distribution table is given to us and we need to find the range and coefficient of range of the given data.

Now, the formula for Range is given as:
$Range=HighestvalueofFrequency-LowestvalueofFrequency$
So, according to the question, from the frequency distribution table we can conclude that,
The Highest value of Frequency is 08 which belongs to the interval 30-40 and the lowest frequency value is 02 which belongs to the interval 0-10.
Then Range will now be given as:
$$\begin{gathered} Range=HighestvalueofFrequency-LowestvalueofFrequency \\ =08-02 \\ =06 \end{gathered}$$
So, Range = 6

Next, we need to calculate the coefficient of range
Now, Coefficient of Range is given as:
$CoefficientofRange={\displaystyle \frac{Highestvalueoffrequency-Lowestvalueoffrequency}{Highestvalueoffrequency+Lowestvalueoffrequency}}...........(i)$
Now, as already concluded earlier from the table of data The Highest value of Frequency is 08 which belongs to the interval 30-40 and the lowest frequency value is 02 which belongs to the interval 0-10.
So,
$$\begin{gathered} Highestvalueoffrequency-Lowestvalueoffrequency \\ =08-02 \\ =06................................................(ii) \end{gathered}$$
And,
$$\begin{gathered} Highestvalueoffrequency+Lowestvalueoffrequency \\ =08+02 \\ =10...................................................(iii) \end{gathered}$$
Now, substituting the values from equation (ii) and (iii) in equation (i) we will get:
$$\begin{gathered} CoefficientofRange={\displaystyle \frac{Highestvalueoffrequency-Lowestvalueoffrequency}{Highestvalueoffrequency+Lowestvalueoffrequency}} \\ ={\displaystyle \frac{6}{10}} \\ =0.6 \end{gathered}$$
Therefore, the coefficient of range is 0.6

Hence, the range is 6 and the coefficient of range is 0.6, for the given data.

Note: While calculating the coefficient of range one has to be careful to take the difference as numerator and sum as denominator, if taken otherwise then the value of coefficient will be wrong.