
Can two angles be supplementary without being a linear pair. Give a few examples?
Answer
400.5k+ views
Hint: Two angles are said to be supplementary angles if their sum is equal to
Complete step-by-step answer:
Let us First see what a linear pair is through a diagram.
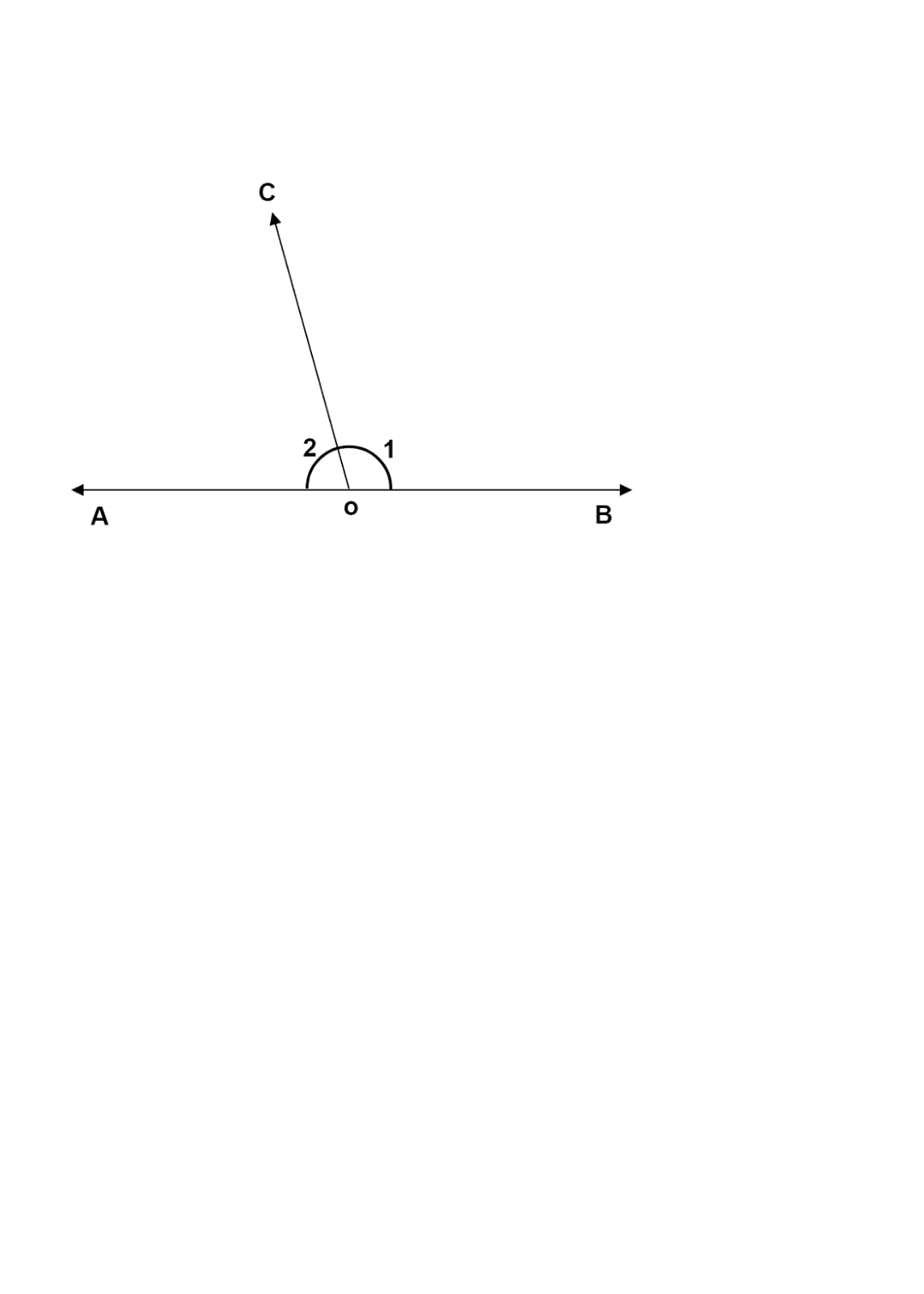
Here
Now, let us look at some other examples where two angles are supplementary angles but they do not form a linear pair.
Let us consider two parallel lines
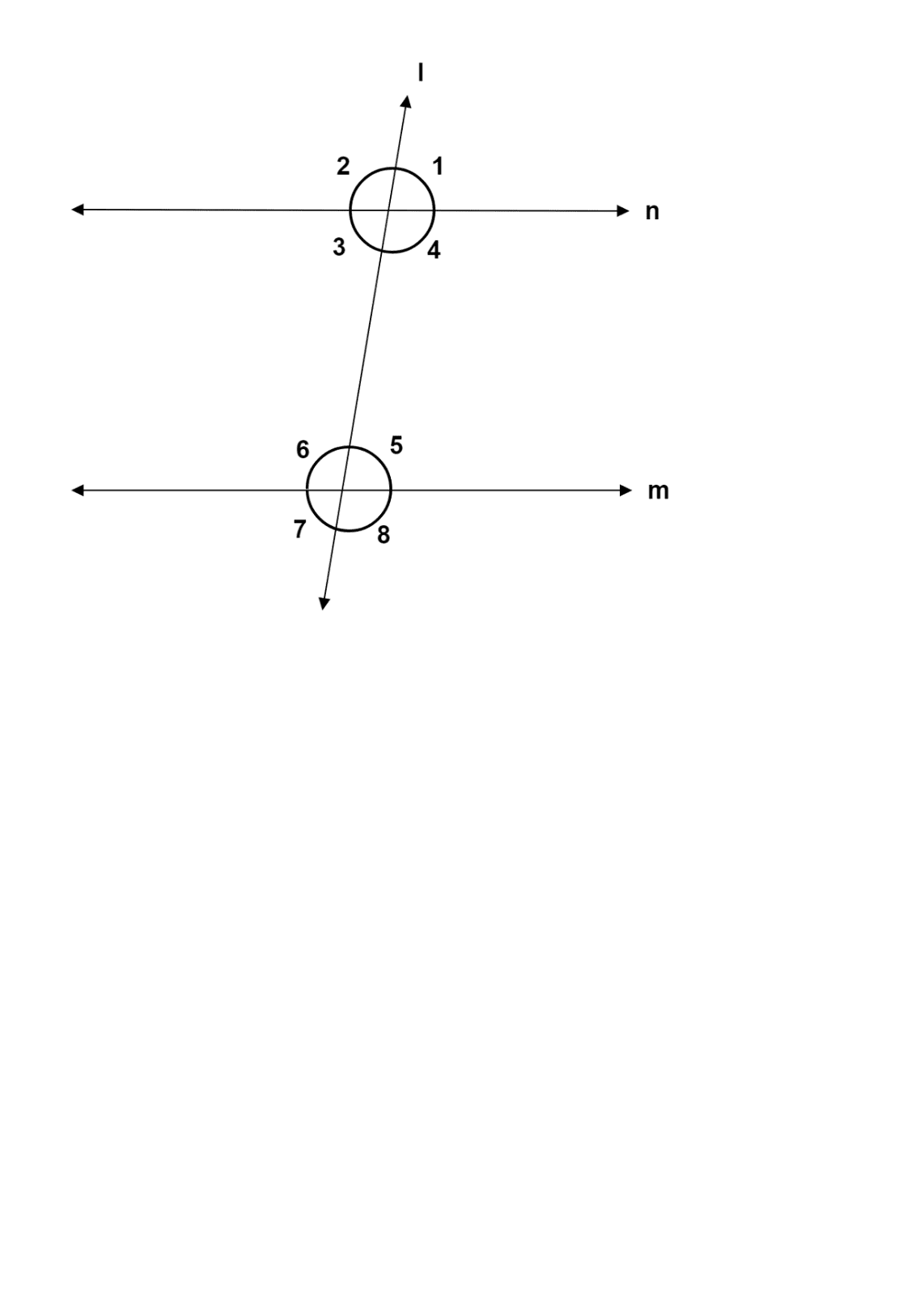
Here,
So, we can say that co-interior angles between two parallel lines are supplementary angles.
Now, let us consider a cyclic quadrilateral. i.e. a quadrilateral whose all vertices lie on a circle circumscribing the quadrilateral.
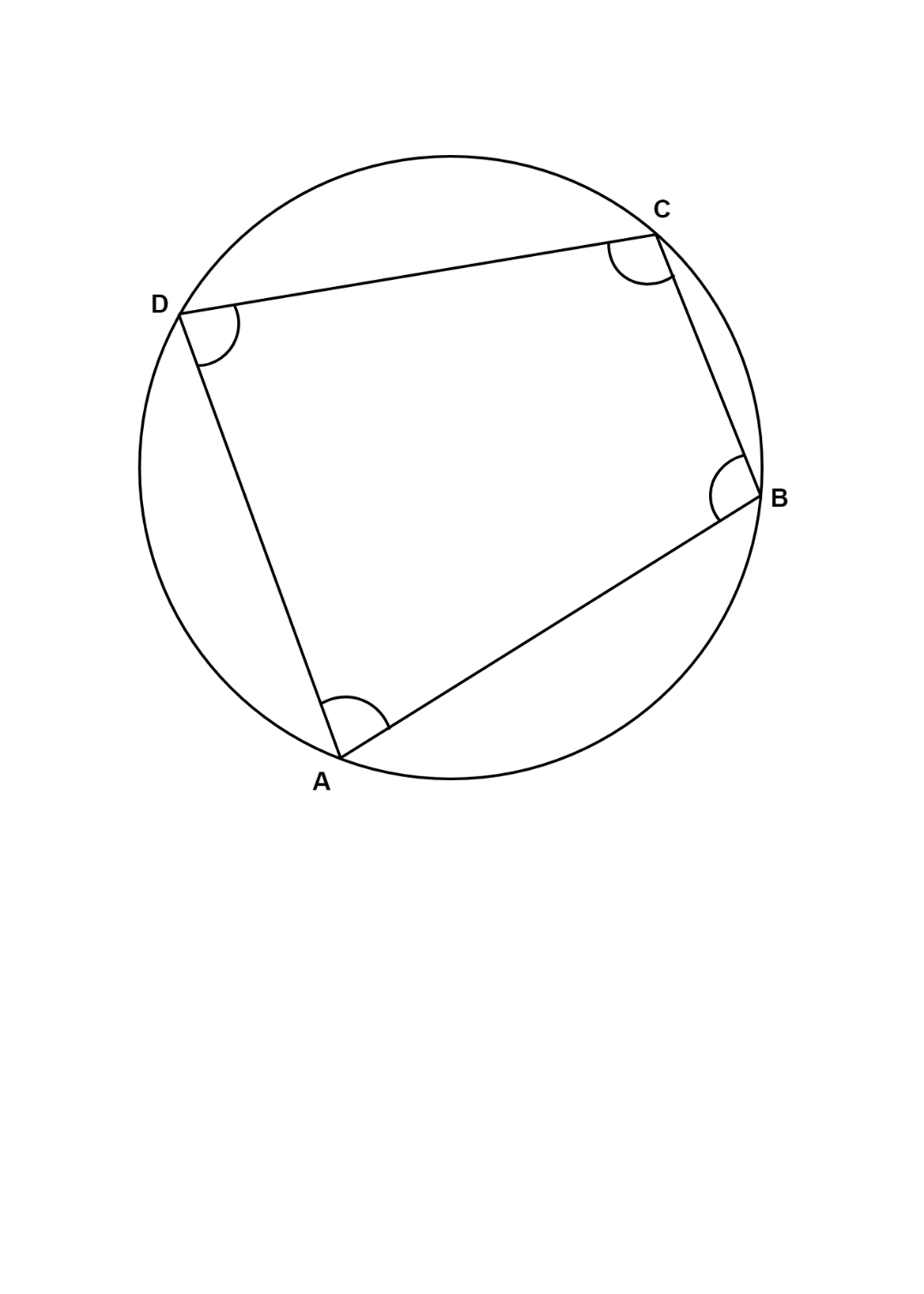
In this cyclic quadrilateral, the opposite angles, i.e.
Hence, we can say that Two angles can be supplementary being a linear pair.
Note: For such types of questions, we need to be very clear with the definitions of every term. Some people get confused between linear pair and supplementary angles and consider that it is a necessary condition for supplementary angles to form a linear pair but they both are different things and we can compare their definitions as well as these things can be best understood using a diagram. Not only these two examples provided above satisfy this but we can find many other examples as well.
Complete step-by-step answer:
Let us First see what a linear pair is through a diagram.

Here
Now, let us look at some other examples where two angles are supplementary angles but they do not form a linear pair.
Let us consider two parallel lines

Here,
So, we can say that co-interior angles between two parallel lines are supplementary angles.
Now, let us consider a cyclic quadrilateral. i.e. a quadrilateral whose all vertices lie on a circle circumscribing the quadrilateral.

In this cyclic quadrilateral, the opposite angles, i.e.
Hence, we can say that Two angles can be supplementary being a linear pair.
Note: For such types of questions, we need to be very clear with the definitions of every term. Some people get confused between linear pair and supplementary angles and consider that it is a necessary condition for supplementary angles to form a linear pair but they both are different things and we can compare their definitions as well as these things can be best understood using a diagram. Not only these two examples provided above satisfy this but we can find many other examples as well.
Recently Updated Pages
Basicity of sulphurous acid and sulphuric acid are

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Trending doubts
In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

List some examples of Rabi and Kharif crops class 8 biology CBSE

How many ounces are in 500 mL class 8 maths CBSE

What is the feminine gender of a stag class 8 english CBSE

Give me the opposite gender of Duck class 8 english CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE





