Question

Can you identify the regular quadrilateral?

Answer 1

Hint: A quadrilateral has four sides, which obviously means four angles. And a regular quadrilateral is a quadrilateral with four equal sides and four equal angles. The sum of all the interior angles of a quadrilateral is 360 degrees so each angle of a regular quadrilateral must be 90 degrees. Opposite sides of a regular quadrilateral must be parallel and the diagonals must bisect each other. So identify the quadrilateral which satisfies all these conditions.

Complete step-by-step answer:
We are asked whether we can identify a regular quadrilateral or not.
A quadrilateral is a polygon with four sides. Some examples of quadrilaterals are rectangle, square, rhombus, parallelogram, trapezium etc.
A regular quadrilateral must have 4 equal sides, 4 equal angles and its diagonal must bisect each other.
Square is the only quadrilateral which satisfies all these conditions.

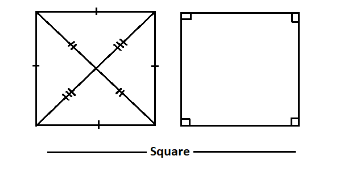
A square is a quadrilateral with all the sides, angles, diagonal equal. The diagonal bisect each other in a square. Therefore, a square is a regular quadrilateral.
A rhombus is a quadrilateral with all the sides equal and its diagonals bisect each other, but all of its angles are not equal.
We already know that only the parallel sides or the opposite sides of a parallelogram are equal. In a trapezium no side is equal to another. A rectangle has only its opposite sides as equal.
Therefore, square is the only regular quadrilateral.
So, the correct answer is “square”.

Note: Do not confuse that rectangle is also a regular quadrilateral. It is not because all the sides are not equal in a rectangle whereas all the angles are equal and diagonals bisect each other. For a quadrilateral to be regular it should have all the sides equal. So the rectangle is not. When all the sides are equal in a rectangle, then it can be considered as a square.