
What is the cartesian form of complex numbers ?
Answer
416.4k+ views
Hint: The cartesian form of a complex number is represented in a two – dimensional plane . Let
Complete step-by-step answer:
A complex number system is a form of number system in which imaginary numbers are represented . The cartesian plane of the complex number has two axes - one is the imaginary axis and the other one is the real axis . For better understanding , let us take an example :
Let
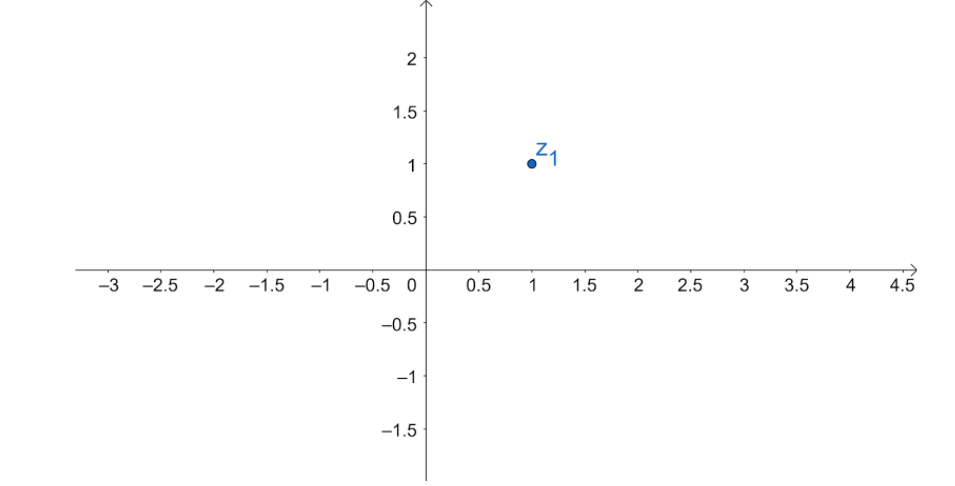
The real number is called the subset of the complex number as when we have
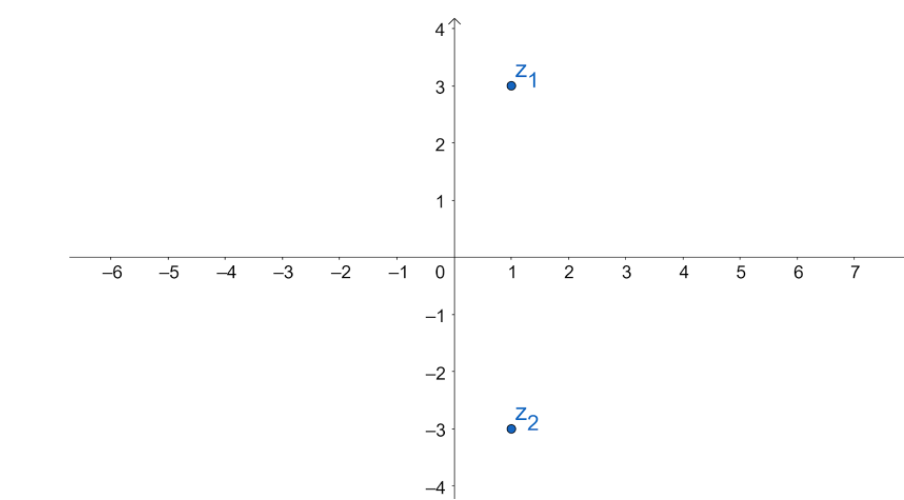
Therefore , the imaginary part will become negative after taking the conjugate of a complex number . A complex number can be represented in a Cartesian axis diagram with a real and an imaginary axis - also known as the Argand diagram .
Note: The argument of a complex number can be calculated by taking
Complete step-by-step answer:
A complex number system is a form of number system in which imaginary numbers are represented . The cartesian plane of the complex number has two axes - one is the imaginary axis and the other one is the real axis . For better understanding , let us take an example :
Let

The real number is called the subset of the complex number as when we have

Therefore , the imaginary part will become negative after taking the conjugate of a complex number . A complex number can be represented in a Cartesian axis diagram with a real and an imaginary axis - also known as the Argand diagram .
Note: The argument of a complex number can be calculated by taking
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

What is the difference between superposition and e class 11 physics CBSE

State and prove Bernoullis theorem class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light




