
Answer
462k+ views
Hint: We should know that when the two triangles are congruent so some congruency theorems are
SSS, SAS, AAS, ASA. Here $A$ denotes the angle and $S$ denote the side. Here when all the three sides are equal then $SSS$ is applicable.
Complete step by step answer:
Here in this questions we are given four options:
A. All three corresponding sides are equal.
B. Two angles and one side between them are congruent.
C. Two angles and the non-included side are congruent
D. Two sides and the angle between them are congruent.
These options are given and we need to explain which option is suitable for the congruency condition.
Here (1)
$SSS$ (side-side-side)
Here the property$SSS$ is given. In the congruency theorem, we know that if $\Delta ABC,\Delta PQR$
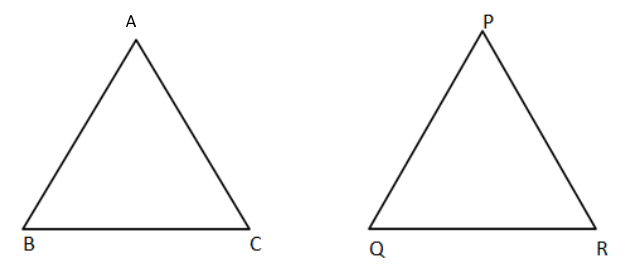
We are given that the three sides are congruent or we are given that
$AB = PQ$
$BC = QR$
$AC = PR$
So we can say that $\Delta ABC \cong \Delta PQR$(by SSS property)
Hence for the SSS property option, A will suit as option A shows all the corresponding sides are congruent which therefore deplete SSS property.
(2) SAS (side-angle-side)
When two angles and one side between them are congruent both triangles are congruent.
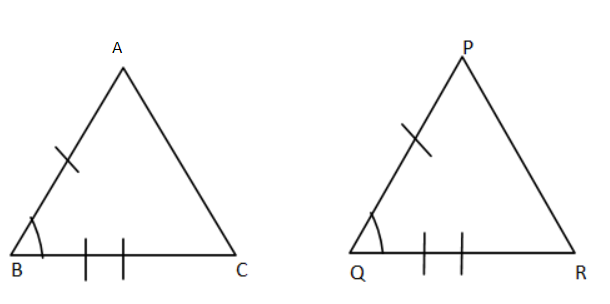
We are given in \[\Delta ABC,\Delta PQR\]
We are given that $AB = PQ$
$BC = QR$ and angle between the sides $AB,BC$ is equal to the angle between $PQ,QR$
$\angle ABC = \angle PQR$
$\Delta ABC \cong \Delta PQR$(SAS property)
Which is the option D
(3) AAS (angle-angle-side)
When two angles and the non-included side are given congruent
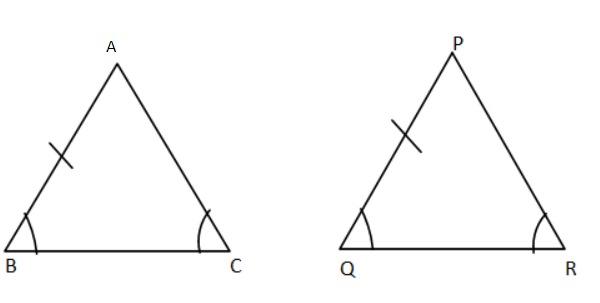 We are given in \[\Delta ABC,\Delta PQR\]
We are given in \[\Delta ABC,\Delta PQR\]
$\angle B = \angle Q$
$\angle C = \angle R$
And the non-included side$AB = PQ$ are equal
Then $\Delta ABC \cong \Delta PQR$(AAS property)
Hence for this the option C is correct.
(4) ASA (angle-side-angle property)
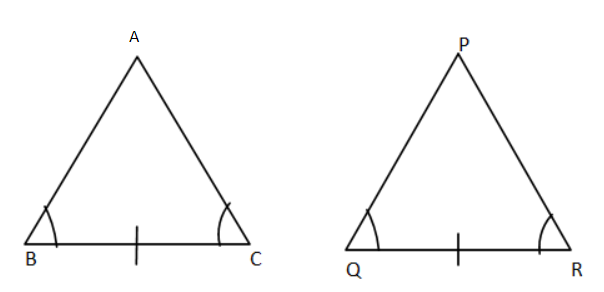
Two angles and the sides between the two angles are given congruent, then the two given triangles are said to be congruent. For example for the triangles \[\Delta ABC,\Delta PQR\]
We are given in \[\Delta ABC,\Delta PQR\]
$\angle B = \angle Q$
$\angle C = \angle R$
$BC = QR$
Then by the given property,
$\Delta ABC \cong \Delta PQR$(ASA property)
So for this option B is the valid one as the two angles and the included sides are equal.
Note:
When we say that if the two triangles are congruent, it means that if we put both the triangles upon each other, then both completely overlap each other. We can consider both the equivalent triangle.
SSS, SAS, AAS, ASA. Here $A$ denotes the angle and $S$ denote the side. Here when all the three sides are equal then $SSS$ is applicable.
Complete step by step answer:
Here in this questions we are given four options:
A. All three corresponding sides are equal.
B. Two angles and one side between them are congruent.
C. Two angles and the non-included side are congruent
D. Two sides and the angle between them are congruent.
These options are given and we need to explain which option is suitable for the congruency condition.
Here (1)
$SSS$ (side-side-side)
Here the property$SSS$ is given. In the congruency theorem, we know that if $\Delta ABC,\Delta PQR$

We are given that the three sides are congruent or we are given that
$AB = PQ$
$BC = QR$
$AC = PR$
So we can say that $\Delta ABC \cong \Delta PQR$(by SSS property)
Hence for the SSS property option, A will suit as option A shows all the corresponding sides are congruent which therefore deplete SSS property.
(2) SAS (side-angle-side)
When two angles and one side between them are congruent both triangles are congruent.
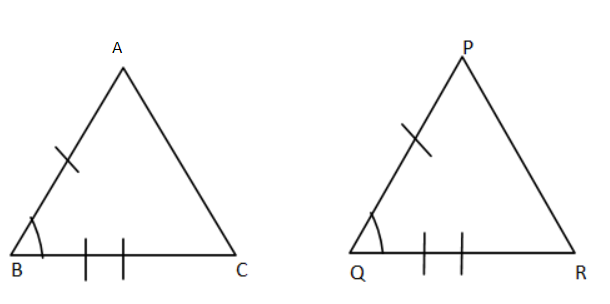
We are given in \[\Delta ABC,\Delta PQR\]
We are given that $AB = PQ$
$BC = QR$ and angle between the sides $AB,BC$ is equal to the angle between $PQ,QR$
$\angle ABC = \angle PQR$
$\Delta ABC \cong \Delta PQR$(SAS property)
Which is the option D
(3) AAS (angle-angle-side)
When two angles and the non-included side are given congruent

$\angle B = \angle Q$
$\angle C = \angle R$
And the non-included side$AB = PQ$ are equal
Then $\Delta ABC \cong \Delta PQR$(AAS property)
Hence for this the option C is correct.
(4) ASA (angle-side-angle property)

Two angles and the sides between the two angles are given congruent, then the two given triangles are said to be congruent. For example for the triangles \[\Delta ABC,\Delta PQR\]
We are given in \[\Delta ABC,\Delta PQR\]
$\angle B = \angle Q$
$\angle C = \angle R$
$BC = QR$
Then by the given property,
$\Delta ABC \cong \Delta PQR$(ASA property)
So for this option B is the valid one as the two angles and the included sides are equal.
Note:
When we say that if the two triangles are congruent, it means that if we put both the triangles upon each other, then both completely overlap each other. We can consider both the equivalent triangle.
Recently Updated Pages
Identify the feminine gender noun from the given sentence class 10 english CBSE

Your club organized a blood donation camp in your city class 10 english CBSE

Choose the correct meaning of the idiomphrase from class 10 english CBSE

Identify the neuter gender noun from the given sentence class 10 english CBSE

Choose the word which best expresses the meaning of class 10 english CBSE

Choose the word which is closest to the opposite in class 10 english CBSE

Trending doubts
Which are the Top 10 Largest Countries of the World?

How do you graph the function fx 4x class 9 maths CBSE

Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Kaziranga National Park is famous for A Lion B Tiger class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

Give 10 examples for herbs , shrubs , climbers , creepers

Write a letter to the principal requesting him to grant class 10 english CBSE




