Question

What is the circumference of the circle if Diameter is 4 cm?

Answer 1

Hint: We are given the diameter of the circle and we have to find the circumference of this circle. Now, diameter is the distance between two points on a circle passing through the center. Hence, the diameter of the circle is 2 times its radius. Using this formula, we can find the radius of the given circle. Now, the circumference of circle is given by the formula
$\text{Circumference} = 2\pi r$

Complete step by step solution:
In this question, we are given a circle and its diameter. We have to find its circumference.
Diameter $ D = 4$cm.
Radius $ \Rightarrow r = $?
Circumference $= $?
Now, first of all let us understand what circle, diameter and radius are.
So, a circle is the collection of all the points lying in a plane at a given distance from the center point.
The distance between the center and all the points on the circle is known as radius.
The distance between two points on the circle passing from the center is known as the diameter of the circle.

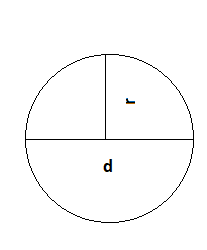
Now, the diameter is double in length as compared to the radius.
So, we can say that diameter of circle is equal to 2 times its radius.
 $ \Rightarrow d = 2r$
Now, we have the diameter as 4 cm and we have to find the radius. Therefore,
$ \Rightarrow 2r = 4$
Divide both sides by 2, we get
$ \Rightarrow r = \dfrac{4}{2}$
$ \Rightarrow r = 2$ cm
Hence, the radius of the circle having diameter 4cm is 2 cm.
Now, circumference of a circle is given by the formula
$ \text{Circumference} = 2\pi r = 2 \times 3.14 \times 2 = 12.56$cm
Hence, the circumference of the circle whose diameter is 4cm is 12.56cm.

Note:
To find the area of the circle, we can use the given two formulas.
1) When diameter is given:
$ \Rightarrow Area = \pi \times \dfrac{{{d^2}}}{4}$
For example: $d = 2$
$ \Rightarrow Area = 3.14 \times \dfrac{{{2^2}}}{4} = 3.14$
2) When radius is given:
$ \Rightarrow Area = \pi \times {r^2}$
For example: $r = 4$
$ \Rightarrow Area = 3.14 \times {4^2} = 50.24$