
How will you compare the emfs of two cells using a potentiometer?
Answer
478.8k+ views
Hint: The emf of two cells can be compared using a potentiometer either by connecting one cell at a time or both cells at a time. You can use the two-way key to connect one cell at a time in a circuit. Obtain the balancing length for each cell separately by placing the jockey on potentiometer wire such that the ammeter shows zero or null deflection. By taking the ratio of both balancing lengths you can compare emfs of two cells.
Formula used:
Complete answer:
Comparison of e.m.f. of the two cells by using a potentiometer can be done by two methods:
1. Individual cell method.
2. Sum and difference method.
The individual cell method is described below.
Make connections as per the following circuit diagram.
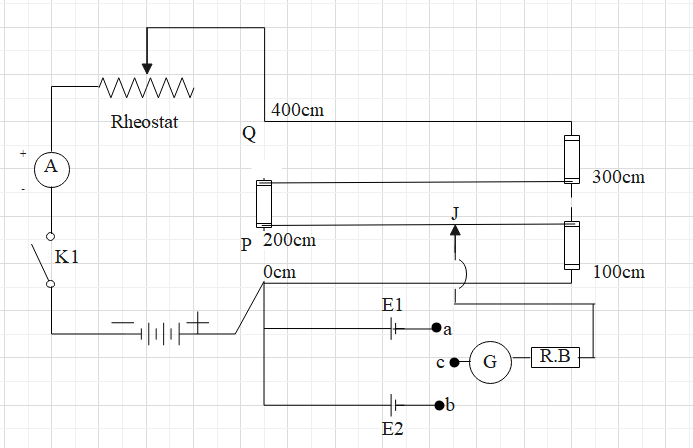
A potentiometer circuit is set up by connecting a battery B, with a key K1 and a rheostat such that point P is at a higher potential than point Q. The cells whose emfs
where
Now, terminal c is tapped towards terminal b and terminal a is kept open. The cell of emfs
From the above two equations, we get
Thus, we can compare the emfs of the two cells. If anyone of the emfs is known, the other can be determined.
Note:
The Potentiometer is one such device that does not draw any current from the circuit and it acts as an ideal voltmeter. It is generally used to determine e.m.f.s of the unknown cell, compare the e.m.f of two cells and to determine the internal resistance of a cell. Do not slide the jockey over the potentiometer wire.
Formula used:
Complete answer:
Comparison of e.m.f. of the two cells by using a potentiometer can be done by two methods:
1. Individual cell method.
2. Sum and difference method.
The individual cell method is described below.
Make connections as per the following circuit diagram.

A potentiometer circuit is set up by connecting a battery B, with a key K1 and a rheostat such that point P is at a higher potential than point Q. The cells whose emfs
where
Now, terminal c is tapped towards terminal b and terminal a is kept open. The cell of emfs
From the above two equations, we get
Thus, we can compare the emfs of the two cells. If anyone of the emfs is known, the other can be determined.
Note:
The Potentiometer is one such device that does not draw any current from the circuit and it acts as an ideal voltmeter. It is generally used to determine e.m.f.s of the unknown cell, compare the e.m.f of two cells and to determine the internal resistance of a cell. Do not slide the jockey over the potentiometer wire.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




