Question

Consider a uniform spherical charge distribution of radius ${R_1}$ centred at the origin O. In this distribution, a spherical cavity of radius ${R_2}$​, centre at P with distance OP=$\alpha $=​${R_1}$−${R_2}$ is made. If the electric field inside the cavity at position $\mathop r\limits^ \to $ is $\mathop E\limits^ \to $ ($\mathop r\limits^ \to $), then the correct statement(s) is (are)

A. $\mathop E\limits^ \to $ is uniform, its magnitude is independent of R2 but its direction depends on $\mathop r\limits^ \to $
B. $\mathop E\limits^ \to $ is uniform, its magnitude depends on R2 and its direction depends on $\mathop r\limits^ \to $
C. $\mathop E\limits^ \to $ is uniform, its magnitude is independent of $\mathop \alpha \limits^ \to $but its direction depends on $\mathop \alpha \limits^ \to $
D. $\mathop E\limits^ \to $ is uniform and both its magnitude and direction depend on $\mathop \alpha \limits^ \to $

Answer 1

Hint: In order to find the electric field we use integration usually. But if integration over the body becomes complicated then we use a simple law called Gauss law in order to find out the electric field due to the charged body. Integration for non uniform shaped bodies will be difficult. Then gauss law might help. We solve this problem using Gauss law.

Formula used:
$\int {E.dA} = \dfrac{{{q_{in}}}}{{{\varepsilon _0}}}$

Complete step by step answer:
There are some limitations where we can apply gauss law. In order to apply gauss law we will consider a gaussian surface. Now that gaussian surface should not pass through the point charge or discrete charge. It can pass through continuous charge. The electric field on that gaussian surface will be due to the charges inside and outside the gaussian surface, but the charge we take for the application of the gauss law should be the charge inside the gaussian surface.
We can take any gaussian surface. But if we need to find an electric field at the point on gaussian surface then the conditions to be followed is, electric field but be same everywhere on the gaussian surface and electric field must be either parallel or perpendicular to gaussian surface and the gaussian surface must be symmetrical.
Gauss law will be
$\int {E.dA} = \dfrac{{{q_{in}}}}{{{\varepsilon _0}}}$
The cross section area we consider for the spherical charge distribution is spherical surface and area will be $4\pi {r^2}$ and the volume will be $\dfrac{4}{3}\pi {r^3}$.
So the charge enclosed inside will be ${q_{in}} = \rho \left( {\dfrac{4}{3}\pi {r^3}} \right)$
$\int {E.dA} = \dfrac{{{q_{in}}}}{{{\varepsilon _0}}}$
$\eqalign{
  & \Rightarrow E\left( {4\pi {r^2}} \right) = \dfrac{{\rho \left( {\dfrac{4}{3}\pi {r^3}} \right)}}{{{\varepsilon _0}}} \cr
  & \Rightarrow \mathop E\limits^ \to = \dfrac{\rho }{{3{\varepsilon _0}}}\mathop r\limits^ \to \cr} $

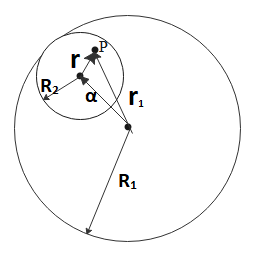
The electric field at point P due to the total sphere will be $\mathop {{E_{{r_1}}}}\limits^ \to $ and the electric field at the point P due to the cavity will be $\mathop {{E_r}}\limits^ \to $
So the electric field at the point P due to the remaining portion of the charged sphere will be $\mathop {{E_{{r_1}}}}\limits^ \to - \mathop {{E_r}}\limits^ \to $
From the vector law we can clearly see that
$\eqalign{
  & \mathop \alpha \limits^ \to + \mathop r\limits^ \to = \mathop {{r_1}}\limits^ \to \cr
  & \Rightarrow \mathop \alpha \limits^ \to = \mathop {{r_1}}\limits^ \to - \mathop r\limits^ \to \cr} $
So the remaining electric field will be $\mathop {{E_{{r_1}}}}\limits^ \to - \mathop {{E_r}}\limits^ \to $
$\eqalign{
  & \mathop {{E_{{r_1}}}}\limits^ \to - \mathop {{E_r}}\limits^ \to = \dfrac{\rho }{{3{\varepsilon _0}}}\mathop {{r_1}}\limits^ \to - \dfrac{\rho }{{3{\varepsilon _0}}}\mathop r\limits^ \to \cr
  & \Rightarrow \mathop {{E_{{r_1}}}}\limits^ \to - \mathop {{E_r}}\limits^ \to = \dfrac{\rho }{{3{\varepsilon _0}}}\left( {\mathop {{r_1}}\limits^ \to - \mathop r\limits^ \to } \right) \cr
  & \Rightarrow \mathop {{E_{{r_1}}}}\limits^ \to - \mathop {{E_r}}\limits^ \to = \dfrac{\rho }{{3{\varepsilon _0}}}\left( {\mathop \alpha \limits^ \to } \right) \cr} $
Hence $\mathop E\limits^ \to $ is uniform and both its magnitude and direction depend on $\mathop \alpha \limits^ \to $
Hence option D will be correct.

Note: Electric field is uniform in the sense, electric field throughout the cavity will be the same and it doesn’t depend on the position vector of the point from the center of the cavity. We can consider the gaussian surface of any shape. But in order to calculate the electric field very easily by avoiding the long integrations we will generally select a symmetrical gaussian surface.