Question

Consider two ‘postulates’ given below:
(i)Given any two distinct points A and B, there exists a third point C, which is in between A and B.
(ii)There exist at least three points that are not on the same line.
Do these postulates contain any undefined terms? Are there postulates consistent? Do they follow from Euclid’s postulates? Explain.

Answer 1

Hint: Here, we will first use the postulates contain undefined terms, which are point and line and consistent means that there are no contradiction between the two postulates, that is, if they deal with two different situation to check both the postulates are consistent. Then we will compare these postulates with Euclid’s postulates.

Complete step-by-step answer:
We are given that these are two postulates.
We know that postulates are the basic structure from which lemmas and theorems are derived.
Yes, these postulates contain undefined terms, which are point and line.
We know that consistency means that there are no contradictions between the two postulates, that is, if they deal with two different situations.
We will now see what both postulates mean.
We will first draw the line with any two distinct points A and B on it.

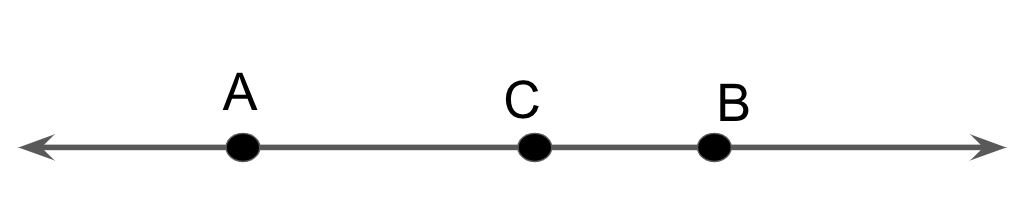
We can say that the given two points A and B, there is a point C lying on the line in between
them.
Draw the line with two points A and B and take the point C, which is not on the line.

We said that given A and B, we can take C not lying on the line through A and B.

Since both their postulates say two different things, so these are consistent.
We know that Euclid's postulates talk about straight lines drawn from one point to another, terminated line, circle, right-angle and two straight lines intersecting.
Since we know both these postulates are not related to Euclid’s postulates.
Thus, these postulates do not follow from Euclid’s postulates.

Note: While solving these types of questions, students should know that a statement is an axiom, which is taken to be true without proof and postulates are the basic structure from which lemmas and theorems are derived. We need to know about the Euclid’s postulates before finding the solution of the problem.