
Construct a regular pentagon in a circle of radius
Answer
467.1k+ views
Hint: Here, we will first draw a circle with the given radius. Now we will draw the regular pentagon by drawing the central angle of a pentagon with the help of the radii of the circle. We will further construct the regular pentagon by using one side of the pentagon which is drawn using the central angle.
Complete step-by-step answer:
First, we will draw a circle of radius,
The center of this circle is at point
Now, we will draw two radii
We have divided the angle by 5 because in a pentagon, we have 5 sides and hence, 5 angles.
Now, we will join
After joining them together, we will consider
Now, with
Similarly, we will consider
Joining
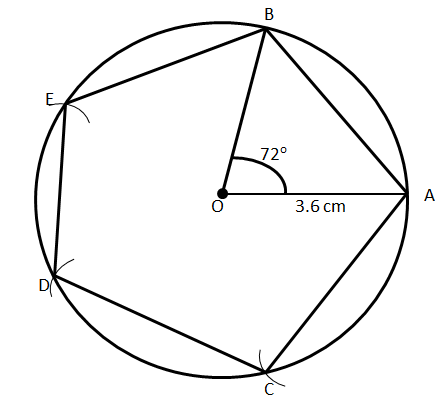
Hence, this is the required regular pentagon inscribed in a circle of radius
Thus, this is the required answer.
Note: In geometry, a pentagon is a polygon which has five sides. Hence, it is known as a five-sided polygon. In order to calculate the sum of interior angles of any polygon, we use the formula
Thus, the sum of interior angles of a five-sided polygon is:
Now, in the case of a regular pentagon, we have all the sides of same length and all the angles of equal measure.
Thus, each angle in a regular pentagon is of
Also, the central angle of a regular pentagon is of
Complete step-by-step answer:
First, we will draw a circle of radius,
The center of this circle is at point
Now, we will draw two radii
We have divided the angle by 5 because in a pentagon, we have 5 sides and hence, 5 angles.
Now, we will join
After joining them together, we will consider
Now, with
Similarly, we will consider
Joining

Hence, this is the required regular pentagon inscribed in a circle of radius
Thus, this is the required answer.
Note: In geometry, a pentagon is a polygon which has five sides. Hence, it is known as a five-sided polygon. In order to calculate the sum of interior angles of any polygon, we use the formula
Thus, the sum of interior angles of a five-sided polygon is:
Now, in the case of a regular pentagon, we have all the sides of same length and all the angles of equal measure.
Thus, each angle in a regular pentagon is of
Also, the central angle of a regular pentagon is of
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
List some examples of Rabi and Kharif crops class 8 biology CBSE

How many ounces are in 500 mL class 8 maths CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

Advantages and disadvantages of science

10 slogans on organ donation class 8 english CBSE




