Question

Construct a square whose diagonal is 6cm. Measure the side. Find also its area.

Answer 1

Hint- Here we will proceed by drawing the given diagonal and then finding the centre of the square which will give us all sides of the required square, with the help of scale and compass.

Complete step-by-step answer:
Now we have given that,
The length of the diagonal is 6cm.
And we have to construct a square.
Steps of construction are-
1) Draw the rough diagram and mark the given measures.

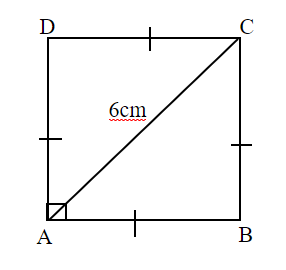
2) Draw a line segment AC=6cm

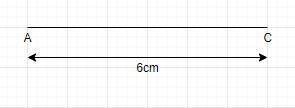
3) Draw a perpendicular bisector XY of AC.

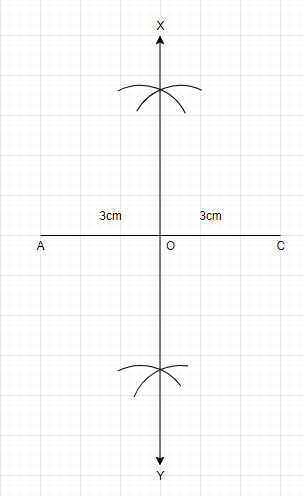
4) XY intersects AC at O. We get OC=OA=3cm.

5) With O as centre, draw two arcs of radius 3cm cutting the line XY at points B and D.

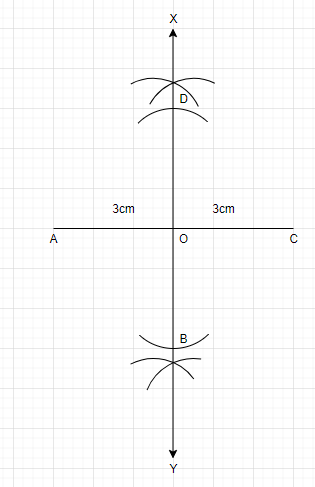
6) Join AB, BC, CD and DA.

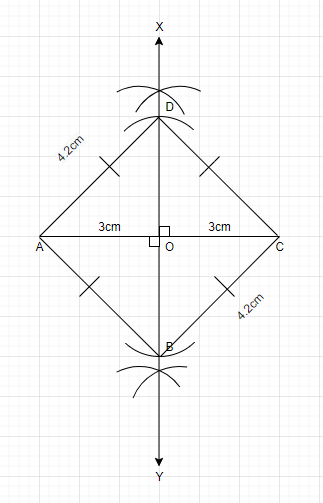
Thus in this way, we construct a square.
Now we have to Measure its side. We can measure that by using a ruler.
Or AB=BC=CD=DA=4.2cm
Now also we have to find the area of the square.
We know that area of square =$a^2$
Here a=4.2cm
Thus, the area of square =${4.2}^2$
 =17.64$c{m}^2$

Note- In this particular problem, we have obtained the midpoint M of the diagonal AC by operating the ends of the compass more than half of the length of diagonal AC ( i.e. the distance between the ends of the compass is more than 3.2cm) and then by placing the fixed end at point at A and then at point B respectively, these arcs will intersect at two distinct points. Join these lines by a dotted line and this dotted line will intersect the diagonal AC at the midpoint of AC i.e. point M.