
Construct rectangle JUMP with the following measurements. Find its area.
Answer
433.2k+ views
Hint: We are given the question we have to construct a rectangle with the given measurements. First we will choose a base for our rectangle. It can be any one of the given measurement in the question We will take one of them as the base, then with the help of protractor we will then construct a right angle with the line segment of the side equal to the other measurement and then keep on making the right angles with given measurements alternatively until we joint back with the given base. Also we have to calculate the area of the given rectangle measurements. The bigger value in the measurement will be considered as the length and the other one as the breadth , then we will use the formula stated below to find the area of the rectangle,
We will then have calculated the area as well as constructed the rectangle of the given measurements.
Complete step-by-step answer:
First steps for the construction of the rectangle.
We will choose
We will then make a line perpendicular (
Then we will again make a line perpendicular (
Now our three sides are done, then we will make a fourth line perpendicular (
Your diagram should look like the diagram below :
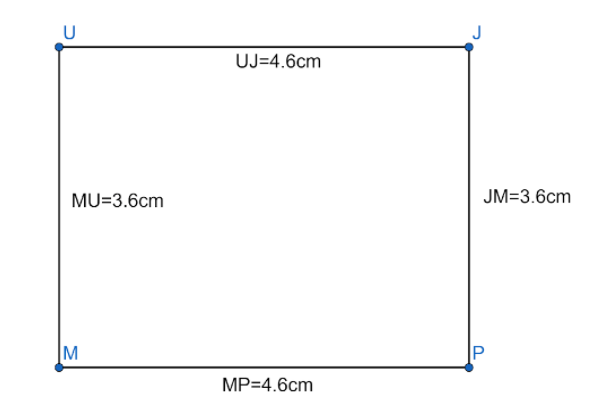
Now we will calculate the area of this rectangle.
The base
Which is the required answer.
So, the correct answer is “
Note: Remember the formula for the area of a rectangle,
Also remember that the rectangle has equal diagonals but those diagonals although bisecting each other are not perpendicular to each other. The perimeter of the rectangle is calculated using the formula,
Where
We will then have calculated the area as well as constructed the rectangle of the given measurements.
Complete step-by-step answer:
First steps for the construction of the rectangle.
We will choose
We will then make a line perpendicular (
Then we will again make a line perpendicular (
Now our three sides are done, then we will make a fourth line perpendicular (
Your diagram should look like the diagram below :

Now we will calculate the area of this rectangle.
The base
Which is the required answer.
So, the correct answer is “
Note: Remember the formula for the area of a rectangle,
Also remember that the rectangle has equal diagonals but those diagonals although bisecting each other are not perpendicular to each other. The perimeter of the rectangle is calculated using the formula,
Where
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
List some examples of Rabi and Kharif crops class 8 biology CBSE

What is the feminine gender of a stag class 8 english CBSE

How many ounces are in 500 mL class 8 maths CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

How many ten lakhs are in one crore-class-8-maths-CBSE

Advantages and disadvantages of science





