
Construct the following angles using set squares: 75 degree
Answer
481.5k+ views
Hint: Set squares are the thin flat triangular shaped scales having one angle of 90 degree. To draw an angle of 75 degree, the two different set squares having 30 degree and 45 degree are going to be used simultaneously. So that
Complete step-by-step answer:
Step1 use both the set squares in combinations as
Step 2 As there are two angles of 45 degree in 45 degree set square. It can be placed in two different ways and a ray can be drawn from either side measuring 45 degrees at one vertex.
Step 3 Place the
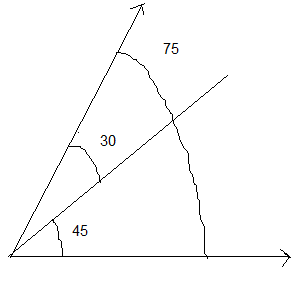
Step 4 Now the ray so obtained is the other aim of the angle required.
Note: The set squares are the two thin flat triangular scales. One having angles
We constructed the angle of 75 degrees with the help of both set squares at the same time. The set squares are used to construct the angles having combinations of
The set squares are also used to draw parallel lines as well as the perpendicular lines. Set squares are also used to draw different types of geometrical shapes like squares, rectangles and certain types of triangles as well.
Complete step-by-step answer:
Step1 use both the set squares in combinations as
Step 2 As there are two angles of 45 degree in 45 degree set square. It can be placed in two different ways and a ray can be drawn from either side measuring 45 degrees at one vertex.
Step 3 Place the

Step 4 Now the ray so obtained is the other aim of the angle required.
Note: The set squares are the two thin flat triangular scales. One having angles
We constructed the angle of 75 degrees with the help of both set squares at the same time. The set squares are used to construct the angles having combinations of
The set squares are also used to draw parallel lines as well as the perpendicular lines. Set squares are also used to draw different types of geometrical shapes like squares, rectangles and certain types of triangles as well.
Recently Updated Pages
Basicity of sulphurous acid and sulphuric acid are

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Trending doubts
Full Form of IASDMIPSIFSIRSPOLICE class 7 social science CBSE

The southernmost point of the Indian mainland is known class 7 social studies CBSE

Convert 200 Million dollars in rupees class 7 maths CBSE

How many crores make 10 million class 7 maths CBSE

Write a pair of integers whose sum gives i Zero ii class 7 maths CBSE

List of coprime numbers from 1 to 100 class 7 maths CBSE





