
Copper crystallises into a fcc lattice with edges length
Answer
482.7k+ views
Hint: We know that density is the mean mass of a unit volume of a substance.
We must remember that an appointment of atoms in crystals during which the atomic centers are disposed in space is such that one atom is found at each of the corners of the cube and one at the middle of every face.
We know that in FCC, there are eight atoms at each corner (each shared by 8 unit cells) and six at the faces (each shared by two unit cells).
The number of atoms per unit cell in FCC is
Formula Used:The formula of density is given as below,
Where,
Z = No. of atoms in unit cell
M = Atomic mass of the substance
a = Edge length
Complete step by step answer:
Given data contains,
In FCC, the no. of atoms in per unit cell is 4. Z=4
M
a
Avogadro’s number
The arrangement of copper atoms shows a face-centered-cubic unit cell has four atoms per unit cell.
Let us calculate density by using the formula as,
Substituting the given values in the formula we get,
On simplifying we get,
Finally, the density value is approximately equal to
Note: Also we know that Primitive lattice or simple in which there are points at all the corners of the unit cell. The number of atoms per units cell in a primitive lattice is
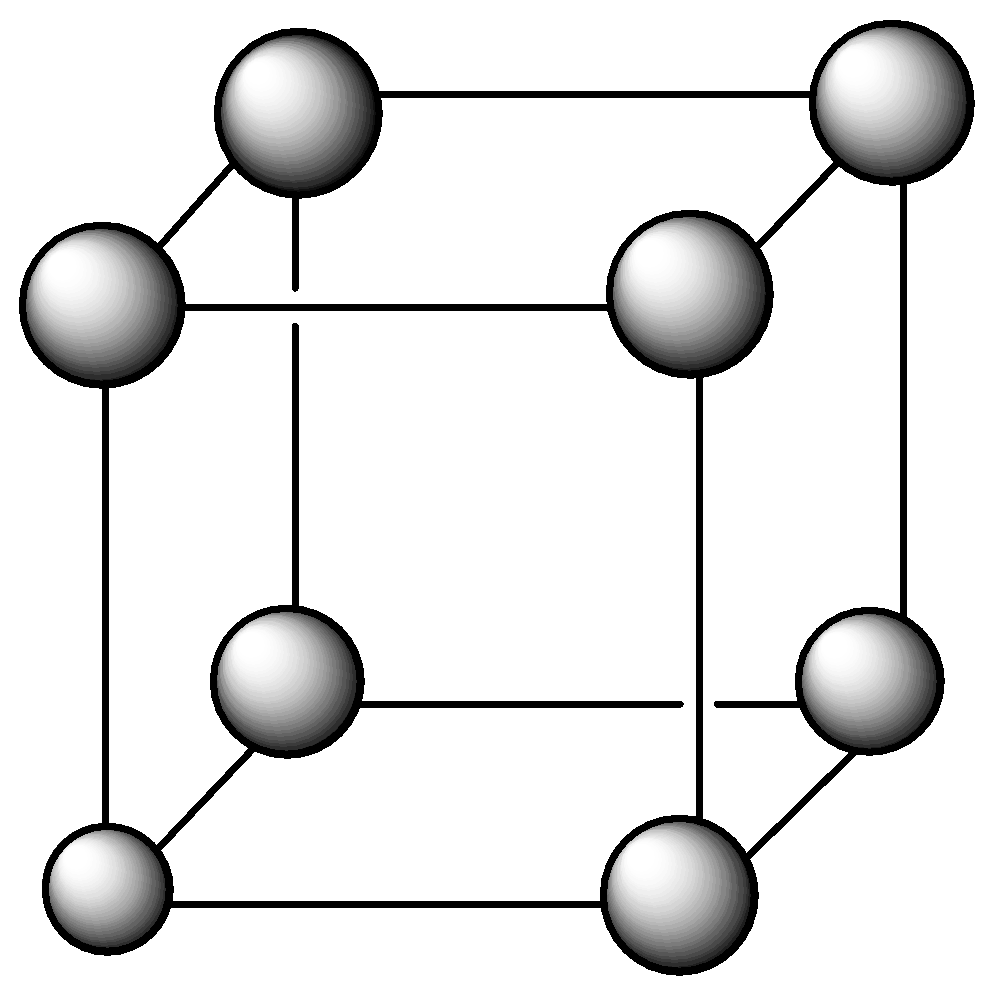
Body-centered lattice in which there are points at all corners as well as in the center of the unit cell. The number of atoms per unit cell is
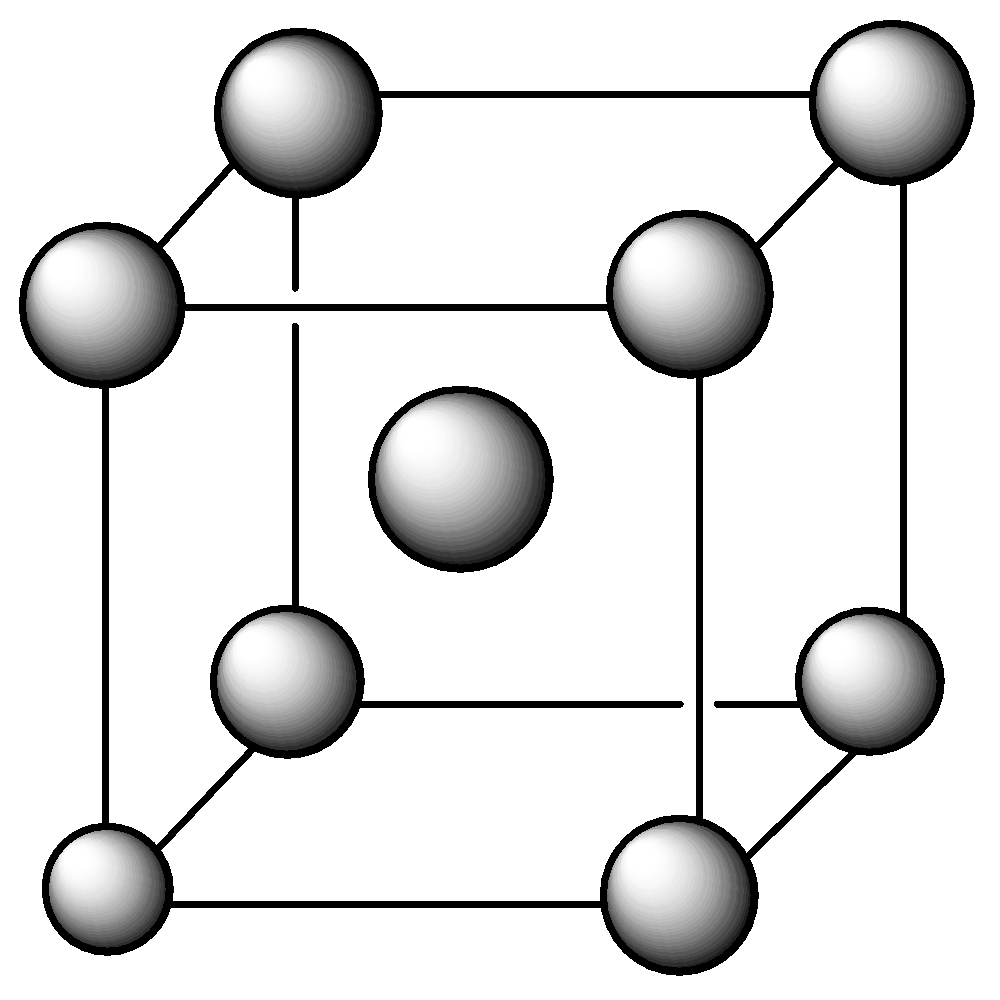
We must remember that an appointment of atoms in crystals during which the atomic centers are disposed in space is such that one atom is found at each of the corners of the cube and one at the middle of every face.
We know that in FCC, there are eight atoms at each corner (each shared by 8 unit cells) and six at the faces (each shared by two unit cells).
The number of atoms per unit cell in FCC is
Formula Used:The formula of density is given as below,
Where,
Z = No. of atoms in unit cell
M = Atomic mass of the substance
a = Edge length
Complete step by step answer:
Given data contains,
In FCC, the no. of atoms in per unit cell is 4. Z=4
M
a
Avogadro’s number
The arrangement of copper atoms shows a face-centered-cubic unit cell has four atoms per unit cell.
Let us calculate density by using the formula as,
Substituting the given values in the formula we get,
On simplifying we get,
Finally, the density value is approximately equal to
Note: Also we know that Primitive lattice or simple in which there are points at all the corners of the unit cell. The number of atoms per units cell in a primitive lattice is

Body-centered lattice in which there are points at all corners as well as in the center of the unit cell. The number of atoms per unit cell is

Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Why is steel more elastic than rubber class 11 physics CBSE

Explain why a There is no atmosphere on the moon b class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE




