Question

What is the critical value of ${{Z}_{\alpha /2}}$ that corresponds to a 94% confidence?

Answer 1

Hint: We solve this problem by calculating the percentage of area that represents the critical value of ${{Z}_{\alpha /2}}$ that corresponds to a 94% confidence. We use the formula to calculate the value of $\alpha $ that is,
$\left( 1-\alpha \right)\times 100=\text{Confidence level}$
Then the area to the left that represents the critical value of ${{Z}_{\alpha /2}}$ that corresponds to a 94% confidence is given as
$A=\left( 1-\dfrac{\alpha }{2} \right)$
Then we find the Z – score that corresponds to the area. To find the Z – scores we need to check the horizontal and vertical values and add them to get the Z – score corresponding to that area. We need to note that the Z – scores for areas greater than 0.5 are positive.


Complete step-by-step solution:

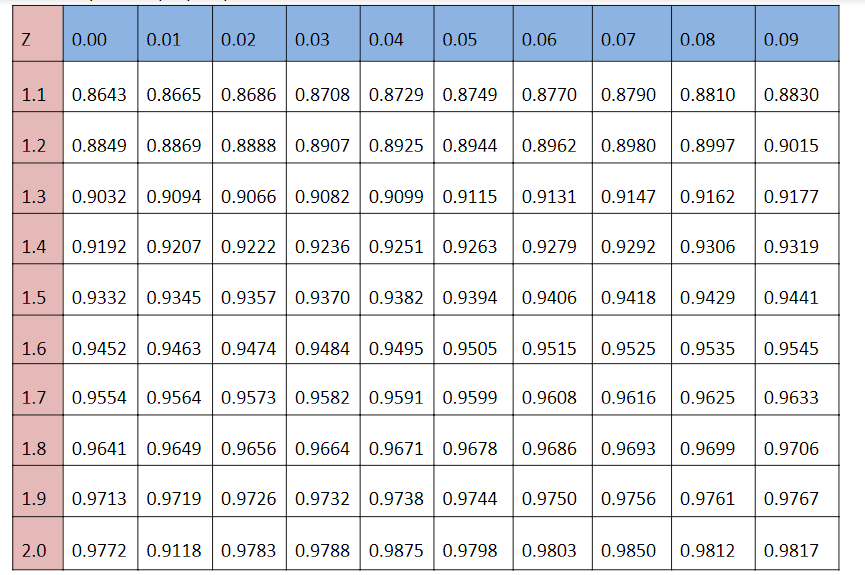
We are given that the confidence level as 94%
Now, let us find the value of $\alpha $
We know that the formula used to calculate the value of $\alpha $ is given as,
$\left( 1-\alpha \right)\times 100=\text{Confidence level}$
By substituting the required values in above formula we get,
$\begin{align}
  & \Rightarrow \left( 1-\alpha \right)\times 100=94 \\
 & \Rightarrow 1-\alpha =0.94 \\
 & \Rightarrow \alpha =0.06 \\
\end{align}$
Now, let us find the area that corresponds to the critical value of ${{Z}_{\alpha /2}}$ that corresponds to a 94% confidence is given as
$A=\left( 1-\dfrac{\alpha }{2} \right)$
By using the above formula we get the required area as,
$\begin{align}
  & \Rightarrow A=1-\dfrac{0.06}{2} \\
 & \Rightarrow A=1-0.03=0.97 \\
\end{align}$
Now, let us find the Z – score that corresponds to the area of 0.97.
Let us assume that the required Z – score as $'z'$
Here, we can see that the table used for standard conversions of area under graph to Z – score does not include 0.97.
But we can see that there is an area equal to 0.9699 which is very close to the required area 0.97.
We know that we need to add horizontal and vertical values of Z – scores to get the Z – score corresponding to that respective area.
We know that for the area greater than 0.5 the Z – score will be positive.
So, we can say that the Z – score for 0.9699 is given as,
$\begin{align}
  & \Rightarrow z=1.8+0.08 \\
 & \Rightarrow z=1.88 \\
\end{align}$
Here, we can see that the value of Z – score corresponding to area 0.97 is 1.88.
Therefore, we can conclude that the critical value of ${{Z}_{\alpha /2}}$ that corresponds to 94% confidence is given as 1.88 that is,
$\Rightarrow {{Z}_{0.06/2}}={{Z}_{0.03}}=1.88$

Note: We need to note that if the area given is greater than 0.5 then the Z – score should be always positive. So, we need positive Z – scores from the table corresponding to the assumed areas.
Also we need to note that we take the Z – score of area equal to 0.97 as the Z – score of area equal to 0.9699 because they are almost equal. It is having very little difference.
But in the same case if the area is having some more difference then we need to use the interpolation theorem to find the required Z – score.