
Define angle of friction.
Answer
433.4k+ views
1 likes
Hint : The coefficient of friction between two surfaces is the tangent of the angle at which the object overcomes friction force and starts sliding. It can also be defined as the tangent of the angle of friction.
Complete step by step answer:
When a body experiences a friction force
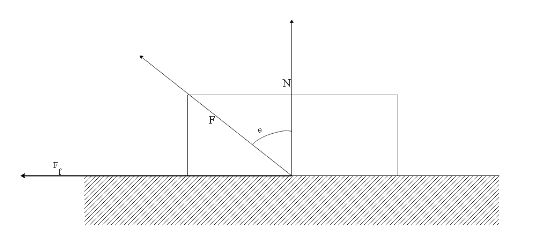
The coefficient of friction
Now from the schematic diagram of the block, we can calculate the value of
Equalizing equation (1) and (2), we can write
Hence the angle of friction is such that the tangent of the angle of friction is the coefficient of friction. If the external force overcomes the friction force acting on the object, then the object will start moving and the friction force will be constant irrespective of the movement of the block once it starts moving.
Note:
Since the coefficient friction of any surface can only lie between 0 and 1, the angle of friction can lie only between
Complete step by step answer:
When a body experiences a friction force

The coefficient of friction
Now from the schematic diagram of the block, we can calculate the value of
Equalizing equation (1) and (2), we can write
Hence the angle of friction is such that the tangent of the angle of friction is the coefficient of friction. If the external force overcomes the friction force acting on the object, then the object will start moving and the friction force will be constant irrespective of the movement of the block once it starts moving.
Note:
Since the coefficient friction of any surface can only lie between 0 and 1, the angle of friction can lie only between
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

Try out challenging quizzes on this topic
made by experts!
made by experts!
Take me there!



