
Define elastic collision. Show that in one dimensional elastic collision of two bodies, the relative velocity of separation after collision is equal to the relative velocity of approach before collision.
Answer
429k+ views
2 likes
Hint: In any collision the linear momentum is conserved so the momentum before the collision and the momentum after the collision will be equal. Also in elastic collisions the kinetic energy is also conserved. So using these conditions the relation between the relative velocity of approach and relative velocity of separation can be calculated.
Formula used:
The momentum of a body of mass
And the kinetic energy is
Complete answer:
A collision is said to occur between two bodies, either if they collide against each other or if the path of one is affected by the force exerted by the other. The collisions between the particles are of two types.
(1)Elastic Collision: If there is no loss of kinetic energy during a collision then the collision is called elastic collision. In elastic collision the kinetic energy and the momentum is conserved.
Examples: Collision between subatomic particles , collision between glass balls etc.
(2)Inelastic collision: If there is loss of kinetic energy during a collision then the collision is called inelastic collision. In inelastic collisions the kinetic energy is not conserved.
Examples: Collision between two vehicles, Collision between a ball and floor.
As shown in figure consider two perfectly elastic body A and B of masses
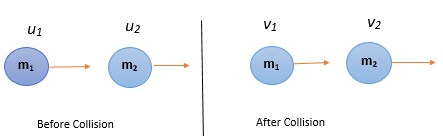
As the linear momentum is conserved in any collision so the linear momentum before the collision will be equal to linear momentum after the collision.
The linear momentum of A before the collision is
The linear momentum of B before the collision is
The linear momentum of A after the collision is
The linear momentum of B after the collision is
So according to conservation of linear momentum
Also the kinetic energy is conserved in elastic collisions. So
The kinetic energy of A before the collision is
The kinetic energy of B before the collision is
The kinetic energy of A after the collision is
The kinetic energy of B after the collision is
So according to conservation of linear momentum the kinetic energy before the collision is equal to the kinetic energy after the collision . so,
But from conservation of linear momentum we got
So dividing these two equations we get
So the relative velocity of A with respect to B before the collision = Relative velocity of B with respect to A after the collision.
Or this can also be said that the relative velocity of approach is equal to the relative velocity of separation.
So this is proved that in one dimensional elastic collision of two bodies, the relative velocity of separation after collision is equal to the relative velocity of approach before collision.
Note:
When two bodies of equal masses suffer one dimensional elastic collision , their velocities will get exchanged. Also when an elastic body collides against another body of equal mass, initially at rest, after the collision the first body comes to rest while the second body moves with the initial velocity of the first.
When a light body collides against a massive body at rest , the light body rebounds after the collision with equal, and opposite velocity while the massive body remains at rest..
Formula used:
The momentum of a body of mass
And the kinetic energy is
Complete answer:
A collision is said to occur between two bodies, either if they collide against each other or if the path of one is affected by the force exerted by the other. The collisions between the particles are of two types.
(1)Elastic Collision: If there is no loss of kinetic energy during a collision then the collision is called elastic collision. In elastic collision the kinetic energy and the momentum is conserved.
Examples: Collision between subatomic particles , collision between glass balls etc.
(2)Inelastic collision: If there is loss of kinetic energy during a collision then the collision is called inelastic collision. In inelastic collisions the kinetic energy is not conserved.
Examples: Collision between two vehicles, Collision between a ball and floor.
As shown in figure consider two perfectly elastic body A and B of masses

As the linear momentum is conserved in any collision so the linear momentum before the collision will be equal to linear momentum after the collision.
The linear momentum of A before the collision is
The linear momentum of B before the collision is
The linear momentum of A after the collision is
The linear momentum of B after the collision is
So according to conservation of linear momentum
Also the kinetic energy is conserved in elastic collisions. So
The kinetic energy of A before the collision is
The kinetic energy of B before the collision is
The kinetic energy of A after the collision is
The kinetic energy of B after the collision is
So according to conservation of linear momentum the kinetic energy before the collision is equal to the kinetic energy after the collision . so,
But from conservation of linear momentum we got
So dividing these two equations we get
So the relative velocity of A with respect to B before the collision = Relative velocity of B with respect to A after the collision.
Or this can also be said that the relative velocity of approach is equal to the relative velocity of separation.
So this is proved that in one dimensional elastic collision of two bodies, the relative velocity of separation after collision is equal to the relative velocity of approach before collision.
Note:
When two bodies of equal masses suffer one dimensional elastic collision , their velocities will get exchanged. Also when an elastic body collides against another body of equal mass, initially at rest, after the collision the first body comes to rest while the second body moves with the initial velocity of the first.
When a light body collides against a massive body at rest , the light body rebounds after the collision with equal, and opposite velocity while the massive body remains at rest..
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Class 10 Question and Answer - Your Ultimate Solutions Guide

Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




