
Define how you can draw tangent lines.
Answer
456.3k+ views
Hint: The equation which gives a linear relationship between two variables is termed to be as tangent lines. The instantaneous slope at some
Complete step-by-step answer:
To draw tangent lines, there are three main points to keep in mind.
We have to choose a point on the curve.
At that point on the curve, we have to put the straight edge.
Make sure that the point is equidistant from the curve on both sides of the point by adjusting the angles of the straight edge.
Let us draw a rough sketch of the graph so that the tangent lines become easier to understand.
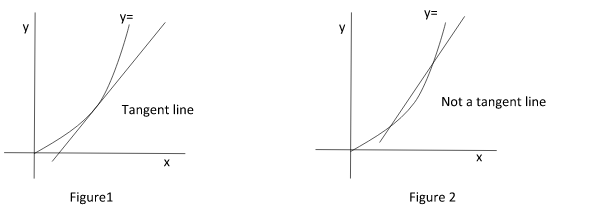
We have drawn a rough sketch of
Note: When we take a closer look to graph we can examine that on zooming it looks more and more linear as we keep zooming in and then, if we draw a straight line that traces appears to be a linear function on zooming and that straight line is the tangent line.
Complete step-by-step answer:
To draw tangent lines, there are three main points to keep in mind.
We have to choose a point on the curve.
At that point on the curve, we have to put the straight edge.
Make sure that the point is equidistant from the curve on both sides of the point by adjusting the angles of the straight edge.
Let us draw a rough sketch of the graph so that the tangent lines become easier to understand.
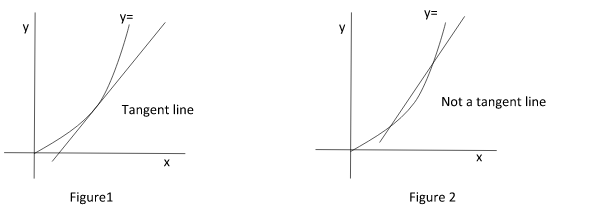
We have drawn a rough sketch of
Note: When we take a closer look to graph we can examine that on zooming it looks more and more linear as we keep zooming in and then, if we draw a straight line that traces appears to be a linear function on zooming and that straight line is the tangent line.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

What is the difference between superposition and e class 11 physics CBSE

State and prove Bernoullis theorem class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light




