
Define oscillatory motion.
Answer
507.9k+ views
Hint: A motion is said to be oscillatory if its diagram in displacement-time graph is either
A. Sinusoidal with constant amplitude. (undamped)
B. Sinusoidal with decreasing amplitude. (damped)
Complete step-by-step answer:
We first need to know about the simple harmonic motion where the force on a body is proportional to its displacement from a mean position. Its equation of motion can be given by,
Where R is the damping force per unit velocity of the object. k is called the force constant. The equation is modified as,
Here,
Undamped oscillatory motion- In this case, R=0
The solution will be,
Where, A is the amplitude of oscillation and
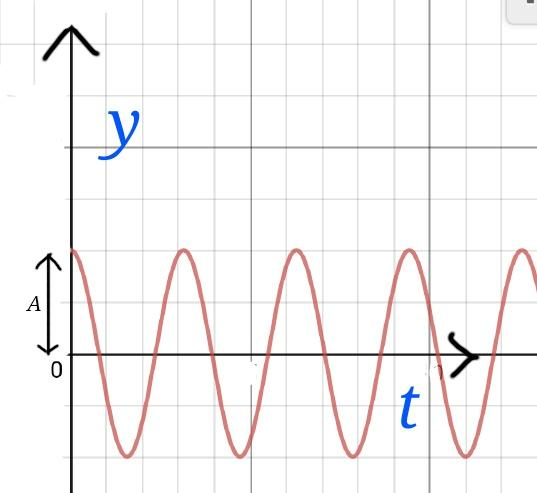
Its energy is given by,
Damped oscillation- In case
If
If
In these two cases there is no oscillation. For oscillation (damped),
In this case, the solution is given by,
Here,
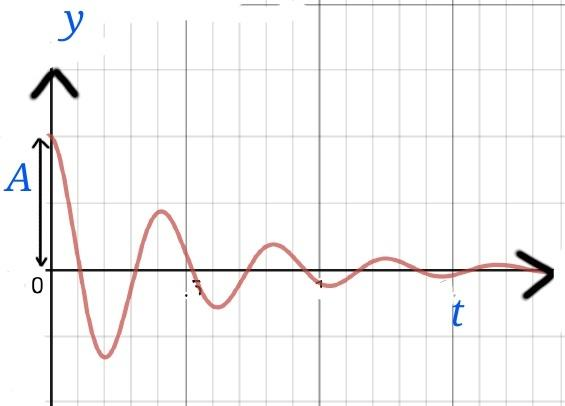
The diagram will look like this.
In this case, the amplitude decreases as ,
Its energy can be shown to be
Note: There is another type of oscillation that is called forced oscillation. In this case, the system is forced to move in oscillation by an oscillatory external force.
A. Sinusoidal with constant amplitude. (undamped)
B. Sinusoidal with decreasing amplitude. (damped)
Complete step-by-step answer:
We first need to know about the simple harmonic motion where the force on a body is proportional to its displacement from a mean position. Its equation of motion can be given by,
Where R is the damping force per unit velocity of the object. k is called the force constant. The equation is modified as,
Here,
Undamped oscillatory motion- In this case, R=0
The solution will be,
Where, A is the amplitude of oscillation and

Its energy is given by,
Damped oscillation- In case
If
If
In these two cases there is no oscillation. For oscillation (damped),
In this case, the solution is given by,
Here,

The diagram will look like this.
In this case, the amplitude decreases as ,
Its energy can be shown to be
Note: There is another type of oscillation that is called forced oscillation. In this case, the system is forced to move in oscillation by an oscillatory external force.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Why is steel more elastic than rubber class 11 physics CBSE

Explain why a There is no atmosphere on the moon b class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE




