Answer
477.6k+ views
Hint- In order to solve this question, we will use the concept of wheatstone bridge. In a wheatstone bridge, the bridge is said to be balanced when the galvanometer shows zero deflection. We will use the condition of zero deflection of the galvanometer to find the unknown value of the resistance and then proceed accordingly.
Complete step-by-step answer:
Condition for balanced bridge-
$\dfrac{{{R_1}}}{{{R_3}}} = \dfrac{{{R_2}}}{{{R_4}}}$
Current sensitivity- The term current sensitivity is defined as when current flow through the galvanometer coil, the coil shows deflection.
Mathematically it can be represented as
${I_s} = \dfrac{\theta }{T} = \dfrac{{nBA}}{c}$
Where n is the number of turns in the coil, B is the magnetic field around the coil, A is the cross sectional area of the coil and c is the restoring torque.
The SI unit of the current sensitivity is radian per ampere.
Now for calculating the value of unknown resistance we will use the condition of balanced bridge.
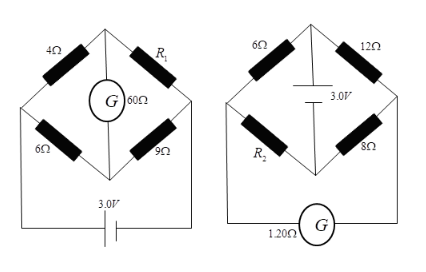
First for the value of resistance ${R_1}$
$\dfrac{{{R_x}}}{{{R_3}}} = \dfrac{{{R_2}}}{{{R_4}}}$
Where ${R_x} = 4,{R_2} = {R_{1,}}{R_3} = 9,{R_4} = 6$
Substituting these values in the above equation
$
\dfrac{{{R_1}}}{9} = \dfrac{4}{6} \\
{R_1} = 6\Omega \\
$
Now for the value of resistance ${R_2}$ from figure second
$\dfrac{{{R_1}}}{{{R_3}}} = \dfrac{{{R_2}}}{{{R_x}}}$
Where ${R_1} = 6,{R_2} = 12,{R_3} = 8,{R_x} = {R_2}$
Substituting these values in the above equation
$
\dfrac{6}{{{R_2}}} = \dfrac{{12}}{8} \\
{R_2} = \dfrac{{6 \times 8}}{{12}} = 4\Omega \\
$
Therefore the ratio of $\dfrac{{{R_1}}}{{{R_2}}}$ is
$\dfrac{{{R_1}}}{{{R_2}}} = \dfrac{6}{4} = \dfrac{3}{2}$
Note- In order to solve questions related to the wheatstone bridge, learn the basic concept behind the wheatstone bridge and also remember the wheatstone bridge is used to find the value of the unknown resistance. It is used to measure the value of the resistance very precisely. Other applications of the wheatstone bridge is ; it is used with operational amplifiers to measure temperature, light, strain etc.
Complete step-by-step answer:
Condition for balanced bridge-
$\dfrac{{{R_1}}}{{{R_3}}} = \dfrac{{{R_2}}}{{{R_4}}}$
Current sensitivity- The term current sensitivity is defined as when current flow through the galvanometer coil, the coil shows deflection.
Mathematically it can be represented as
${I_s} = \dfrac{\theta }{T} = \dfrac{{nBA}}{c}$
Where n is the number of turns in the coil, B is the magnetic field around the coil, A is the cross sectional area of the coil and c is the restoring torque.
The SI unit of the current sensitivity is radian per ampere.
Now for calculating the value of unknown resistance we will use the condition of balanced bridge.
First for the value of resistance ${R_1}$
$\dfrac{{{R_x}}}{{{R_3}}} = \dfrac{{{R_2}}}{{{R_4}}}$
Where ${R_x} = 4,{R_2} = {R_{1,}}{R_3} = 9,{R_4} = 6$
Substituting these values in the above equation
$
\dfrac{{{R_1}}}{9} = \dfrac{4}{6} \\
{R_1} = 6\Omega \\
$
Now for the value of resistance ${R_2}$ from figure second
$\dfrac{{{R_1}}}{{{R_3}}} = \dfrac{{{R_2}}}{{{R_x}}}$
Where ${R_1} = 6,{R_2} = 12,{R_3} = 8,{R_x} = {R_2}$
Substituting these values in the above equation
$
\dfrac{6}{{{R_2}}} = \dfrac{{12}}{8} \\
{R_2} = \dfrac{{6 \times 8}}{{12}} = 4\Omega \\
$
Therefore the ratio of $\dfrac{{{R_1}}}{{{R_2}}}$ is
$\dfrac{{{R_1}}}{{{R_2}}} = \dfrac{6}{4} = \dfrac{3}{2}$
Note- In order to solve questions related to the wheatstone bridge, learn the basic concept behind the wheatstone bridge and also remember the wheatstone bridge is used to find the value of the unknown resistance. It is used to measure the value of the resistance very precisely. Other applications of the wheatstone bridge is ; it is used with operational amplifiers to measure temperature, light, strain etc.
Recently Updated Pages
10 Examples of Evaporation in Daily Life with Explanations

10 Examples of Diffusion in Everyday Life

1 g of dry green algae absorb 47 times 10 3 moles of class 11 chemistry CBSE

What is the meaning of celestial class 10 social science CBSE

What causes groundwater depletion How can it be re class 10 chemistry CBSE

Under which different types can the following changes class 10 physics CBSE

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Which are the Top 10 Largest Countries of the World?

How do you graph the function fx 4x class 9 maths CBSE

Who was the leader of the Bolshevik Party A Leon Trotsky class 9 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Which is the largest saltwater lake in India A Chilika class 8 social science CBSE

Ghatikas during the period of Satavahanas were aHospitals class 6 social science CBSE