
Degree of freedom of a triatomic gas is? (Consider moderate temperature)
A. 6
B. 4
C. 2
D. 8
Answer
507k+ views
2 likes
Hint: Degree of freedom is the number of independent motions a particle can undergo. To find out the degree of freedom we have to find the number of independent motions possible for that particular molecule. Here we have a triatomic gas and in order to find its degree of freedom, we check its possible motions in x, y and z directions.
Complete step by step answer:
We have to find the Degree of freedom of a triatomic molecule. A triatomic gas molecule has 3 atoms in it.
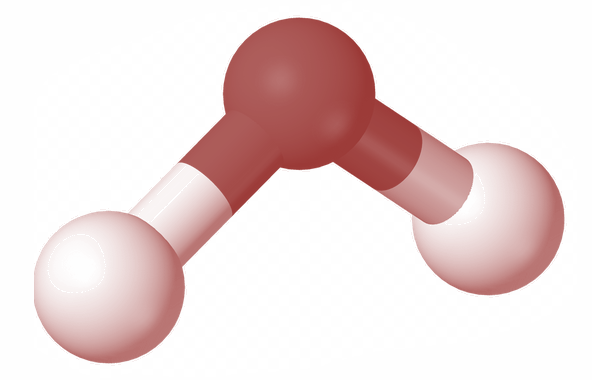
Consider a triatomic gas molecule as shown in the figure above.
We now consider the possible movements of this molecule in the x, y and z axis.
Here this triatomic gas can have a translatory motion along the x, y, and z axis. I.e. triatomic molecules can move along x direction, y direction and z direction.
Hence the translatory degree of freedom of this molecule is 3
Now let us consider the rotational degree of freedom of this molecule.
For that we place two atoms of the molecule on the x axis. Then it can rotate about y axis and z axis. It also has a significant rotation about x axis because here the third atom has a moment of inertia about x axis even if the other two atoms do not have the inertia.
And thus the rotational degree of freedom of this molecule is also three.
Hence the degree of freedom= Translatory degree of freedom + Rotational degree of freedom
=3+3=6
Therefore at moderate temperature the degree of freedom of a triatomic gas equals to 6.
So, the correct answer is “Option A”.
Note: We can also find the degree of freedom by using the general expression
Degree of freedom,
We have a triatomic molecule. And hence the number of particles,
Therefore we have,
Thus the degree of freedom of a triatomic molecule is 6.
Complete step by step answer:
We have to find the Degree of freedom of a triatomic molecule. A triatomic gas molecule has 3 atoms in it.
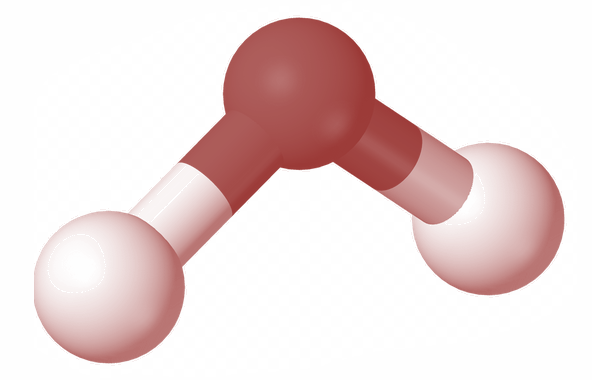
Consider a triatomic gas molecule as shown in the figure above.
We now consider the possible movements of this molecule in the x, y and z axis.
Here this triatomic gas can have a translatory motion along the x, y, and z axis. I.e. triatomic molecules can move along x direction, y direction and z direction.
Hence the translatory degree of freedom of this molecule is 3
Now let us consider the rotational degree of freedom of this molecule.
For that we place two atoms of the molecule on the x axis. Then it can rotate about y axis and z axis. It also has a significant rotation about x axis because here the third atom has a moment of inertia about x axis even if the other two atoms do not have the inertia.
And thus the rotational degree of freedom of this molecule is also three.
Hence the degree of freedom= Translatory degree of freedom + Rotational degree of freedom
=3+3=6
Therefore at moderate temperature the degree of freedom of a triatomic gas equals to 6.
So, the correct answer is “Option A”.
Note: We can also find the degree of freedom by using the general expression
Degree of freedom,
We have a triatomic molecule. And hence the number of particles,
Therefore we have,
Thus the degree of freedom of a triatomic molecule is 6.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




