Question

How many degrees does an obtuse angle have?

Answer 1

Hint:Here, we will classify the types of angles and then find out that an obtuse angle can take the values between which two angles and how many degrees it can have. An angle is formed when two lines intersect at a common point.

Complete step-by-step answer:
In order to answer this question, we should know the difference between all the types of angles.
The meaning of the six types of angles, i.e. acute, obtuse, straight, right, zero, and complete angle is given below:
1. Acute Angle:
An acute angle is an angle that lies between \[0^\circ \]and \[90^\circ \].
Hence, it cannot be equal to \[90^\circ \] i.e. it is always less than the right angle.
Also, it cannot be equal to \[0^\circ \] i.e. there will always be some distance between the two lines forming the angle.

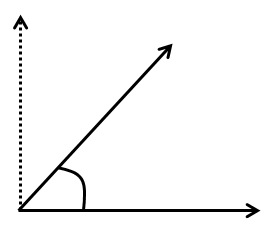
2. Obtuse Angle:
An obtuse angle is an angle that is greater than \[90^\circ \] but less than \[180^\circ \].
Hence, neither it is a straight line nor it forms an ‘L’ shape.

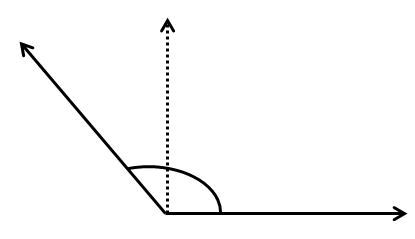
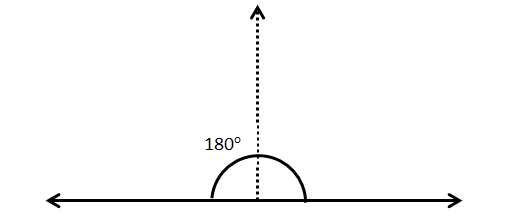
3. Straight Angle:
A straight angle is an angle that is equal to \[180^\circ \], i.e.
It forms a straight line.

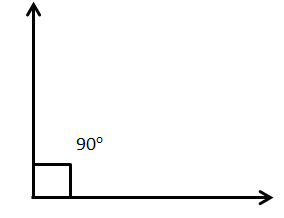
4. Right Angle:
The right angle is an angle that is equal to \[90^\circ \], i.e. it forms an ‘L’ shape.
The two lines making a right angle are called perpendicular lines.

5. Zero Angle:
Zero angles is an angle that is equal to \[0^\circ \].
This angle is formed when the two lines making an angle, lie on top of one another.

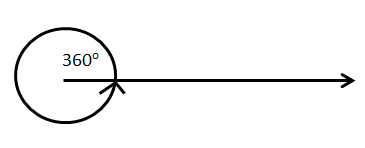
6. Complete Angle:
The complete angle is an angle that is full i.e. the measure of which is \[360^\circ \].

Now, with the help of the above points, we can say that:
An obtuse angle is any angle larger than \[90^\circ \] but less than \[180^\circ \]
Therefore, this is the required answer.

Note: In mathematics, degrees are a unit of angle measure. A full circle is divided into 360 degrees and hence, a quarter of a circle, which forms a right angle is equal to one-fourth of 360 degrees i.e. 90 degrees. A degree has a symbol \[^\circ \] and hence, right angle\[ = 90^\circ \]. Now, another unit to measure angles is called radian. A radian is equal to the amount an angle would have to be open to capture an arc of the circle’s circumference of equal length to the circle’s radius. Hence, \[360^\circ = 2\pi \] radians.