
Derive an expression for the centripetal acceleration of a body moving with constant speed in a circular path.
Answer
434.9k+ views
Hint: Motion in a circular path means that the angular velocity of the particle undergoing circular motion is constant with time. One should always take care of the difference between scalar and vector quantity. Speed is a scalar quantity and velocity is a vector quantity. If the speed is constant, it doesn’t imply that the acceleration is zero but if the velocity is constant, it always implies that acceleration is zero or motion is non-accelerated.
Formula used:
Complete answer:
To understand the statement mathematically, let’s consider a particle undergoing circular motion with speed ‘v’ and radius ‘R’ on a circular track.
Hence, its angular velocity ‘
Let’s see properly marked diagram from top:
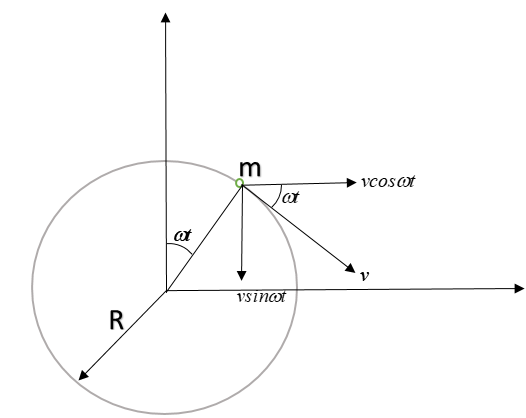
Resolving the speed as velocity into x and y components, so:
To find the acceleration of the particle, let’s differentiate the velocity expression.
=
=
Putting
Hence, vector form of centripetal acceleration is:
Expression for centripetal acceleration of a body undergoing circular motion:
As we derived
To find the magnitude:
Hence centripetal acceleration is
Note:
As we derived the expression for centripetal acceleration of the body undergoing uniform circular motion, we can see that the magnitude of this acceleration is constant with time. One should always remember that whenever a body moves in a curve (basically changes its direction of motion), it will always experience centripetal force acting on it. In short, direction can’t be changed without the application of force. In the above case, the string is providing necessary centripetal force.
Formula used:
Complete answer:
To understand the statement mathematically, let’s consider a particle undergoing circular motion with speed ‘v’ and radius ‘R’ on a circular track.
Hence, its angular velocity ‘
Let’s see properly marked diagram from top:

Resolving the speed as velocity into x and y components, so:
To find the acceleration of the particle, let’s differentiate the velocity expression.
=
=
Putting
Hence, vector form of centripetal acceleration is:
Expression for centripetal acceleration of a body undergoing circular motion:
As we derived
To find the magnitude:
Hence centripetal acceleration is
Note:
As we derived the expression for centripetal acceleration of the body undergoing uniform circular motion, we can see that the magnitude of this acceleration is constant with time. One should always remember that whenever a body moves in a curve (basically changes its direction of motion), it will always experience centripetal force acting on it. In short, direction can’t be changed without the application of force. In the above case, the string is providing necessary centripetal force.
Recently Updated Pages
Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Class 10 Question and Answer - Your Ultimate Solutions Guide

Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




