
Derive the equation of motion with the graphical method.
Answer
439.7k+ views
Hint
We know that the three equations of motion form the backbone of classical mechanics, and have seen the derivation of the equations of motion using the calculus method. One drawback of the calculus method is that you need a different expression for deriving each equation of motion. This drawback is not present in the graphical method as only one velocity-time graph is enough for all the three equations of motion.
Complete step by step answer
The graph of velocity against time is drawn for an object that starts moving with an initial velocity
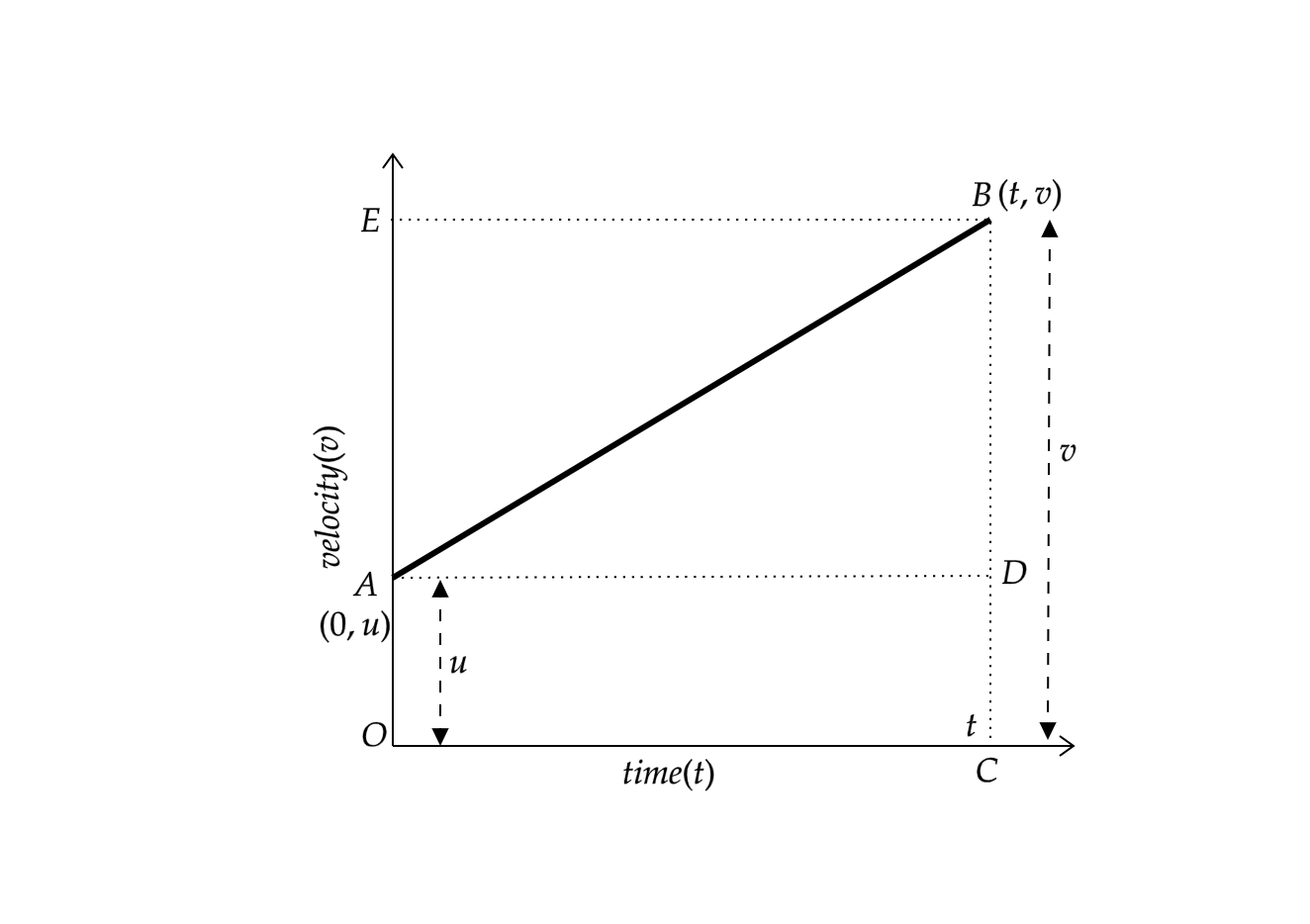
Now we all know that the slope of the curve of a velocity-time graph gives the acceleration of the particle and the area under the curve gives us the displacement of the particle.
The curve or the plot of the motion is given by the line AB; and since the co-ordinates of the points A and B are known, its slope can be given as
Now we know that this slope gives us the value of the acceleration of the particle, hence we can say
This is the first equation of motion. Now the second equation of motion involves the displacement of the particle and we have discussed above that the area under the curve gives the displacement of the particle. Using this approach, we can say that the area of trapezium AOBC gives the displacement. We know that the area of a trapezium is given as half the product of the sum of the parallel sides and the distance between them.
For the given graph, we can say that
Substituting the values of the mentioned sides, we get
Substituting the value of the final velocity from the first equation and simplifying, we get
This is the second equation of motion.
From the first equation of motion, we can say that
Substituting this value of time in equation (a) obtained above, we get
This is the third equation of motion.
Thus we have successfully derived all the equations using the graphical method.
Note
We have three methods of deriving the equations of motion, namely the algebraic method, the graphical method and the calculus method. The algebraic method can be related very closely to the graphical method that we used in our solution above. The calculus method is used when the object changes its state over infinitesimal intervals of time and hence depicting the motion by a graph is not feasible.
We know that the three equations of motion form the backbone of classical mechanics, and have seen the derivation of the equations of motion using the calculus method. One drawback of the calculus method is that you need a different expression for deriving each equation of motion. This drawback is not present in the graphical method as only one velocity-time graph is enough for all the three equations of motion.
Complete step by step answer
The graph of velocity against time is drawn for an object that starts moving with an initial velocity

Now we all know that the slope of the curve of a velocity-time graph gives the acceleration of the particle and the area under the curve gives us the displacement of the particle.
The curve or the plot of the motion is given by the line AB; and since the co-ordinates of the points A and B are known, its slope can be given as
Now we know that this slope gives us the value of the acceleration of the particle, hence we can say
This is the first equation of motion. Now the second equation of motion involves the displacement of the particle and we have discussed above that the area under the curve gives the displacement of the particle. Using this approach, we can say that the area of trapezium AOBC gives the displacement. We know that the area of a trapezium is given as half the product of the sum of the parallel sides and the distance between them.
For the given graph, we can say that
Substituting the values of the mentioned sides, we get
Substituting the value of the final velocity from the first equation and simplifying, we get
This is the second equation of motion.
From the first equation of motion, we can say that
Substituting this value of time in equation (a) obtained above, we get
This is the third equation of motion.
Thus we have successfully derived all the equations using the graphical method.
Note
We have three methods of deriving the equations of motion, namely the algebraic method, the graphical method and the calculus method. The algebraic method can be related very closely to the graphical method that we used in our solution above. The calculus method is used when the object changes its state over infinitesimal intervals of time and hence depicting the motion by a graph is not feasible.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




