
Derive the formula for the volume of a cone, given to you in the figure using the symbols as explained.
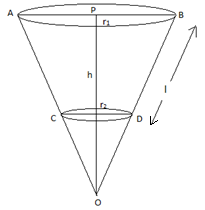
h = height of frustum
l = slant height of frustum

Answer
525k+ views
Hint: We will assume the height of the cone, slant height of the cone OCD also its slant height to be a variable. Then we will write these variables into the given variables in the question. To calculate the volume of frustum, we will subtract the volume of the cone OCD from the volume of the cone AOB.
Complete step-by-step answer:
We have been given the height of the frustum (h), radius of upper part (
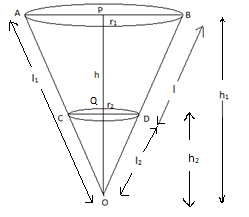
Now let us assume
From the above figure, we can observe that OA = OC + AC.
We can see that OC = OD =
Also, OP = OQ + PQ
As we know that the volume of frustum is equal to the difference between the cone OAB and cone OCD.
We know that if a cone has radius and height r and h respectively, then its volume is as follows:
Now in
As both angles are common to the triangles
Since both angles are
As we know that the corresponding sides of a similar triangle are proportional,
On substituting
On cross multiplication, we get as follows:
So after using the value of
Volume of frustum
We know that
Volume of frustum
Taking
Volume of frustum
We know that
Volume of frustum
Hence the formula for the volume of the frustum of a cone is derived to be
Note: Be careful while calculation and also take care of the algebraic sign as there is a chance of error after submitting the value of
Complete step-by-step answer:
We have been given the height of the frustum (h), radius of upper part (

Now let us assume
From the above figure, we can observe that OA = OC + AC.
We can see that OC = OD =
Also, OP = OQ + PQ
As we know that the volume of frustum is equal to the difference between the cone OAB and cone OCD.
We know that if a cone has radius and height r and h respectively, then its volume is as follows:
Now in
As both angles are common to the triangles
Since both angles are
As we know that the corresponding sides of a similar triangle are proportional,
On substituting
On cross multiplication, we get as follows:
So after using the value of
Volume of frustum
We know that
Volume of frustum
Taking
Volume of frustum
We know that
Volume of frustum
Hence the formula for the volume of the frustum of a cone is derived to be
Note: Be careful while calculation and also take care of the algebraic sign as there is a chance of error after submitting the value of
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




