Question

Derive the relation between Focal length and radius of curvature for spherical mirrors.

Answer 1

Hint: Focal length and radius of curvature directly depends on each other. Focal length and radius of curvature for a mirror have the same unit. Also here we need to apply the rules or laws of reflection.

Complete step-by-step answer:
To find relation between focal length and radius of curvature for spherical mirror
Consider a concave mirror

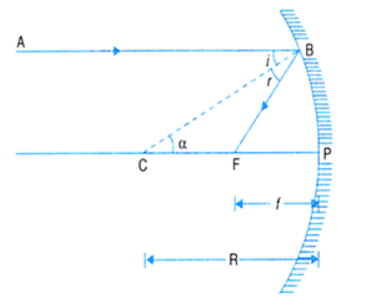
Where,
P = Pole, it is the midpoint of mirror
F = Focal point
C = center of curvature
Cp = Radius of creature
Ray parallel to principal axis strike at point A.
For Applying rules of reflection we have to draw a normal
As we know the line which strikes circular. Also we can say normal at the surface of the sphere will pass through the Center of curvature of mirror or spherical shapes.
Now According to Rules Angle of incident = Angle of Reflection
\[\angle i=\angle r\]
AS OA and OP are parallel to each other
\[\angle \text{ACE}=\text{ }i\text{ }\left( \text{Alternate Angle} \right)\text{ }..........\text{ 1}\]
Also \[\angle \text{FAC}=i\text{ }\left( \text{Reflection Angle} \right)~~~\text{ }\ldots \ldots \ldots \text{ }2\].
By equation 1 and 2
\[\angle \text{ACF}=\angle \text{FAS}=i\]
Therefore CF = FA
When incident way come closer to principal axis then FA= FP
Also we know CF = FP = f (focal length)
Then \[R=CP\]
\[=CF+FP\]
\[=F+F\]
\[=2F\]
So, Radius of curvature is double the focal length.

Note: The Principal focus of a spherical mirror lies in between the role and center of curvature. Also, the Radius of curvature is equal to twice of the focal length.
In general as we know we cut mirrors from the sphere. So the radius of that sphere from which the mirror has cut is known as the radius of curvature of the mirror. There is a focal point for every mirror. So focal length is defined as distance between focal point and mirror.