
Describe the parametric equations of a circle.
Answer
424.8k+ views
Hint: Equation of circle with origin as centre is
Complete step by step answer:
Circle is the locus of a point that moves in the plane such that its distance from a fixed point is always constant. The fixed point is called the centre and the fixed distance is called the radius of the circle.
A parametric equation of a circle is the coordinates of a point on the circle in terms of a single variable. This single variable is called a parameter.
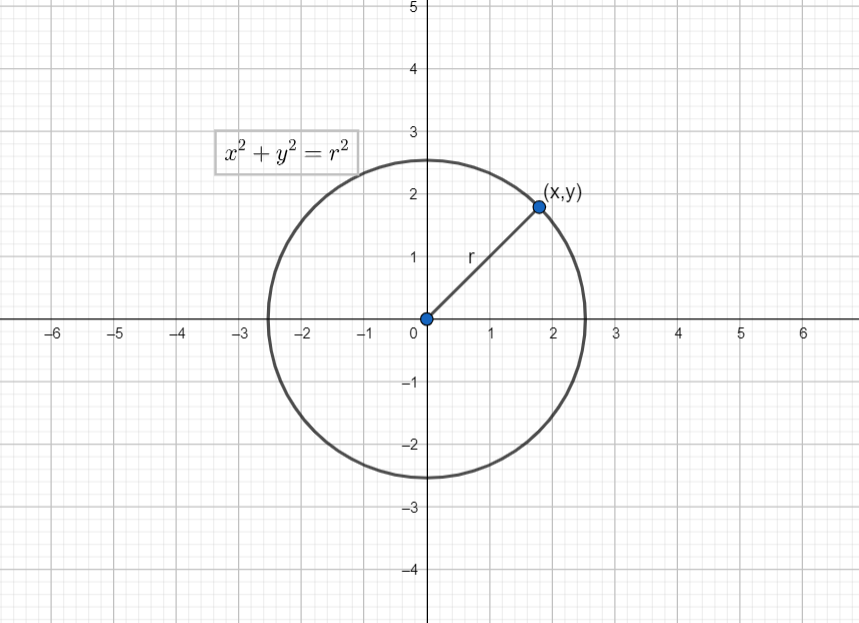
The parametric equation of the circle

Here
From the parametric equation we can find out the coordinates of any point on the circle if we know the radius, centre coordinates, and the radius of the circle.
Note: students should be careful while answering this type of question. This question is completely based upon concepts. So, students should be aware of some basic concepts of mathematics. Many students may have misconception that
Complete step by step answer:
Circle is the locus of a point that moves in the plane such that its distance from a fixed point is always constant. The fixed point is called the centre and the fixed distance is called the radius of the circle.
A parametric equation of a circle is the coordinates of a point on the circle in terms of a single variable. This single variable is called a parameter.

The parametric equation of the circle

Here
From the parametric equation we can find out the coordinates of any point on the circle if we know the radius, centre coordinates, and the radius of the circle.
Note: students should be careful while answering this type of question. This question is completely based upon concepts. So, students should be aware of some basic concepts of mathematics. Many students may have misconception that
Latest Vedantu courses for you
Grade 8 | CBSE | SCHOOL | English
Vedantu 8 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




