
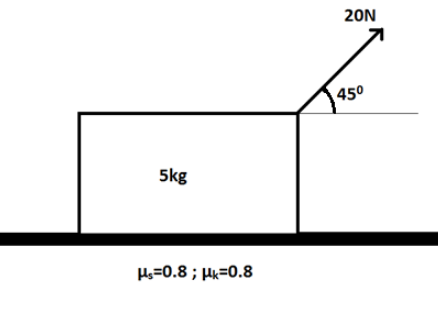
Determine force of limiting friction.

Answer
464.7k+ views
Hint:We know that frictional force is dependent on the coefficient of the friction of the surface and the normal force acting on a body, Thus, we have to find the normal force first which can be done by considering the weight of the block as well as the vertical and horizontal components of the force.
Formulas used:
Where
Where
Complete step by step answer:
As shown in figure, a block of
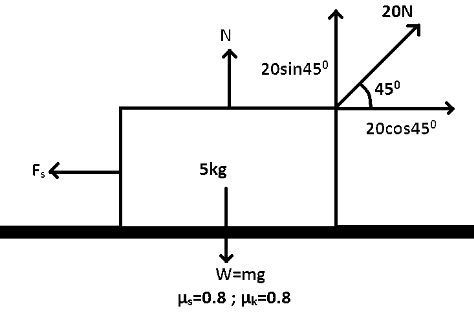
First, we need to find the normal force which can be obtained by considering the vertical forces action on the block in the equilibrium condition.
Normal force and the vertical component of the applied force act in upward direction whereas the weight of the block acts in the downward direction. Therefore,
Now. We know that force of limiting friction
Thus, the answer is the force of limiting friction for the given system is
Note:Here, we have determined the limiting force of friction. Limiting force can be defined as the maximum value of static friction that comes into play when the body is just at the point of sliding over the surface of another body. This is because the amount of friction that can be applied between two surfaces is limited and if the forces acting on the body are made sufficiently great, the motion will occur.
Formulas used:
Where
Where
Complete step by step answer:
As shown in figure, a block of

First, we need to find the normal force which can be obtained by considering the vertical forces action on the block in the equilibrium condition.
Normal force and the vertical component of the applied force act in upward direction whereas the weight of the block acts in the downward direction. Therefore,
Now. We know that force of limiting friction
Thus, the answer is the force of limiting friction for the given system is
Note:Here, we have determined the limiting force of friction. Limiting force can be defined as the maximum value of static friction that comes into play when the body is just at the point of sliding over the surface of another body. This is because the amount of friction that can be applied between two surfaces is limited and if the forces acting on the body are made sufficiently great, the motion will occur.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Why is steel more elastic than rubber class 11 physics CBSE

Explain why a There is no atmosphere on the moon b class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE




