
How do you determine the length of
Answer
444k+ views
Hint: In order to find the solution to the question given above, we use the Law of Sines. It is a relationship between the sides and the angles of an oblique triangle. According to it, the ratio of the length of a side of a triangle to the sine of the angle opposite that side is the same for all the angles and sides in a given triangle.
Formula used:
Law of Sines:
Complete step by step solution:
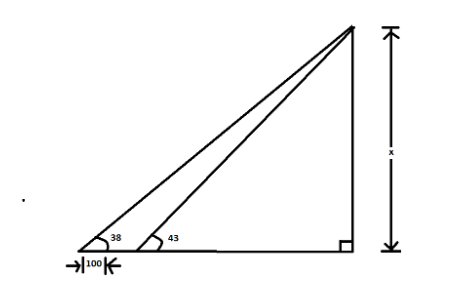
Let the angle at the left-hand side of the
Now, in the oblique triangle, let the last unknown triangle be
Now, we know that in a triangle, the angles are always complementary, so,
Now, to determine the length of the hypotenuse of the right triangle, we will use the Law of Sines.
According to this, let the hypotenuse of the right triangle be
On equating the values, we get,
Now, cross multiple the above equation.
We get,
Now, we can set up our ratio. We now can determine the length of
So, we get that
Our final answer is
So, the correct answer is “
Note: While solving questions similar to the one given above, always remember to use the Law of Sines. With the help of this law you can easily solve the question in no time. The formula for the Law of sines is:
Formula used:
Law of Sines:
Complete step by step solution:

Let the angle at the left-hand side of the
Now, in the oblique triangle, let the last unknown triangle be
Now, we know that in a triangle, the angles are always complementary, so,
Now, to determine the length of the hypotenuse of the right triangle, we will use the Law of Sines.
According to this, let the hypotenuse of the right triangle be
On equating the values, we get,
Now, cross multiple the above equation.
We get,
Now, we can set up our ratio. We now can determine the length of
So, we get that
Our final answer is
So, the correct answer is “
Note: While solving questions similar to the one given above, always remember to use the Law of Sines. With the help of this law you can easily solve the question in no time. The formula for the Law of sines is:
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




