
How do you determine the linear function whose graph is a line that contains the points
Answer
468.6k+ views
Hint: The equation of a line that passes through two points can be calculated by using the two-point form of the equation of a line. We will substitute the given two points into the two-point form of a line. Then after simplifying we can determine the required linear function.
Formula used:
The two-point form of a line passing through the points
Complete step-by-step answer:
As we know we can determine the equation of a line that passes through two points by using the two-point form of a line.
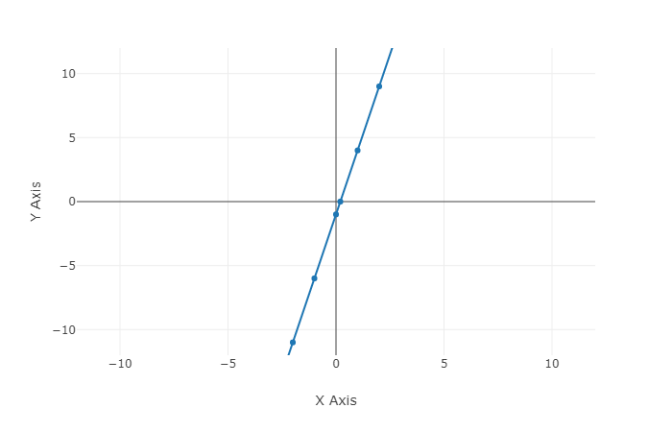
Now, the two given points are
Now, we know that the first coordinate is the x-coordinate and the second coordinate is the y-coordinate.
Here,
For point
For point
Now, as we know that, the two-point form of a line is
Now, we can determine the linear function by substituting the
After substituting the values, we get
Now, after opening the brackets of numerators as well as denominators on both sides, we get
On Right-hand side, simplifying by dividing
Now, by cross multiplying, we get
Now, by subtracting
∴
Note:
There is an alternate way to prove the two points form of the equation of a straight line. Consider the point-slope form of the equation of a line,
we have,
Since the line is passing through the point
Formula used:
The two-point form of a line passing through the points
Complete step-by-step answer:
As we know we can determine the equation of a line that passes through two points by using the two-point form of a line.

Now, the two given points are
Now, we know that the first coordinate is the x-coordinate and the second coordinate is the y-coordinate.
Here,
For point
For point
Now, as we know that, the two-point form of a line is
Now, we can determine the linear function by substituting the
After substituting the values, we get
Now, after opening the brackets of numerators as well as denominators on both sides, we get
On Right-hand side, simplifying by dividing
Now, by cross multiplying, we get
Now, by subtracting
∴
Note:
There is an alternate way to prove the two points form of the equation of a straight line. Consider the point-slope form of the equation of a line,
we have,
Since the line is passing through the point
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

Raindrops are spherical because of A Gravitational class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Why is steel more elastic than rubber class 11 physics CBSE

Explain why a There is no atmosphere on the moon b class 11 physics CBSE




