
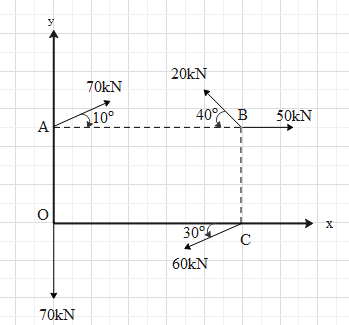
Determine the resultant moment about the origin of the given non-concurrent force system.

Answer
490.8k+ views
Hint: The magnitude of the torque about the origin is equal to the product of force and the perpendicular distance of the origin from the line of force. It is also equal to the product of the distance between the point at which the force is applied and the origin and the force perpendicular to the distance. If the force generates clockwise rotation then the torque is considered to be positive. If the torque generates an anticlockwise rotation then the torque is considered to be negative.
Formula used:
Complete answer:
The torque or moment of force about origin due the a force acting at point is given as
If we talk about the magnitude of torque, then its magnitude is given as
If the force generates clockwise rotation then the torque is considered to be positive. If the torque generates an anticlockwise rotation then the torque is considered to be negative.
Let us first the torque by each force.
Consider the force of 70 kN.
The force perpendicular to
And
Consider the force of 60 kN.
The force perpendicular to
And
Let us find the torque due to the forces at point B.
Before that, resolve the force 20 kN into its horizontal and vertical components. Its horizontal component will be
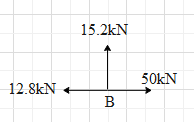
Now the net horizontal force at point B is
And the vertical force is 12.8kN.
Therefore, the torque due to the horizontal force at the B is
The torque due to the vertical force at B is
However, the rotation due to
The torque due the force at point O is zero perpendicular distance is zero.
Therefore, the net torque about the origin is
Note:
The actual formula for the magnitude of the torque about the origin is given as
Formula used:
Complete answer:
The torque or moment of force about origin due the a force acting at point is given as
If we talk about the magnitude of torque, then its magnitude is given as
If the force generates clockwise rotation then the torque is considered to be positive. If the torque generates an anticlockwise rotation then the torque is considered to be negative.
Let us first the torque by each force.
Consider the force of 70 kN.
The force perpendicular to
And
Consider the force of 60 kN.
The force perpendicular to
And
Let us find the torque due to the forces at point B.
Before that, resolve the force 20 kN into its horizontal and vertical components. Its horizontal component will be

Now the net horizontal force at point B is
And the vertical force is 12.8kN.
Therefore, the torque due to the horizontal force at the B is
The torque due to the vertical force at B is
However, the rotation due to
The torque due the force at point O is zero perpendicular distance is zero.
Therefore, the net torque about the origin is
Note:
The actual formula for the magnitude of the torque about the origin is given as
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




