
How do I determine whether a hyperbola opens horizontally or vertically?
Answer
471k+ views
Hint: First we know it is a horizontal or vertical hyperbola.
If it is a horizontal hyperbola since the
That means the curves open left and right.
If it is a vertical hyperbola since the
That means the curves open up and down.
Complete step by step answer:The graph of a hyperbola creates two smooth curves as pictured here:
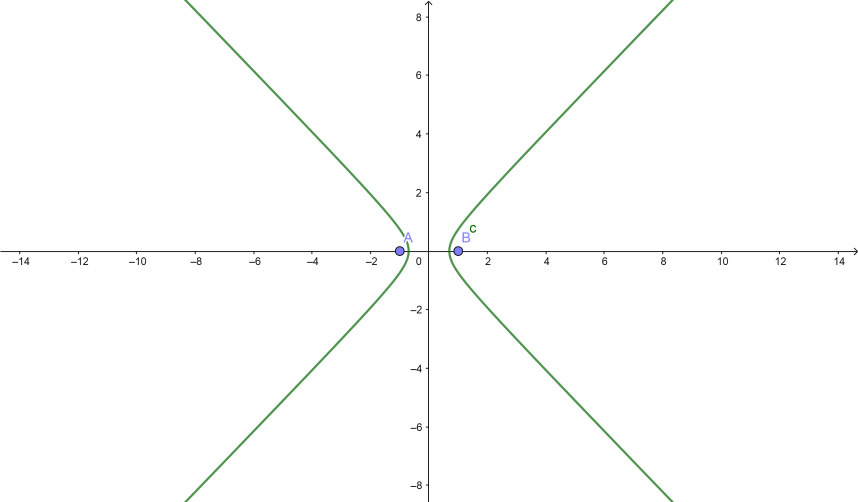
There are two patterns for hyperbolas:
Horizontal:
Vertical:
We can determine the following:
If it is vertical or horizontal:
If the
The horizontal parabola graph is
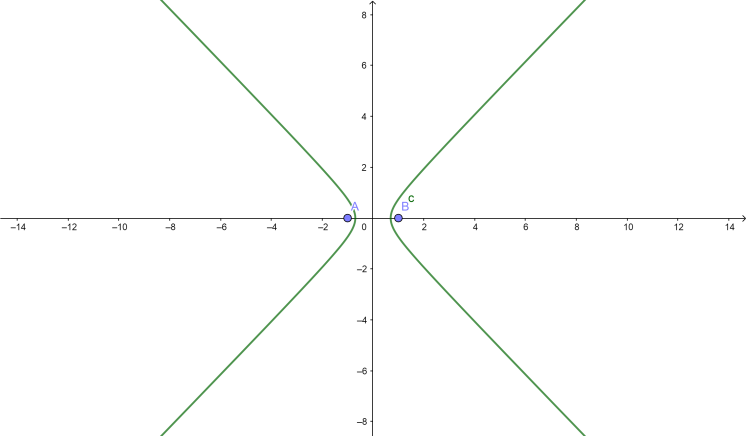
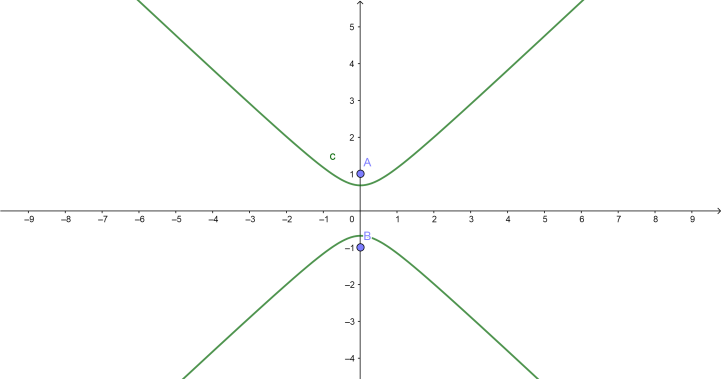
If the
The vertical parabola graph is
The center point as with all conic sections, the center points
The
Note:
Notice that
If
If
If it is a horizontal hyperbola since the
That means the curves open left and right.
If it is a vertical hyperbola since the
That means the curves open up and down.
Complete step by step answer:The graph of a hyperbola creates two smooth curves as pictured here:

There are two patterns for hyperbolas:
Horizontal:
Vertical:
We can determine the following:
If it is vertical or horizontal:
If the
The horizontal parabola graph is

If the
The vertical parabola graph is

The center point as with all conic sections, the center points
The
Note:
Notice that
If
If
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




