Question

Diagonal of a rectangle are equal and perpendicular. Is this statement true? Give reason for your answer?

Answer 1

Hint: In this question, we need to check if the diagonal of a rectangle are equal and perpendicular. For equality we will use the Pythagoras theorem according to which $ {{\left( \text{hypotenuse} \right)}^{2}}={{\left( \text{base} \right)}^{2}}+{{\left( \text{perpendicular} \right)}^{2}} $ is a right angled triangle. For checking perpendicular, we will assume two of them as $ {{90}^{\circ }} $ and contradict our assumption using congruency.

Complete step by step answer:
Here we have to check if diagonals of a rectangle are equal and perpendicular to each other. Let us first draw a rectangle and label it.

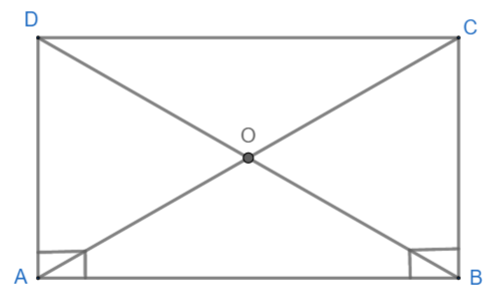
Let us suppose that, we have a rectangle ABCD with diagonals AC and BD. So we first need to prove that AC = BD.
As we know, in a rectangle adjacent sides are perpendicular to each other. Therefore, $ \angle A+\angle B+\angle C+\angle D={{90}^{\circ }} $ .
So from the figure, we can say that, $ \Delta ABC $ is a right-angled triangle with $ \angle B={{90}^{\circ }} $ . So now we can apply Pythagoras theorem in this triangle.
Pythagoras theorem is given as $ {{\left( \text{hypotenuse} \right)}^{2}}={{\left( \text{base} \right)}^{2}}+{{\left( \text{perpendicular} \right)}^{2}} $ .
From $ \Delta ABC $ , $ {{\left( \text{AC} \right)}^{2}}={{\left( \text{AB} \right)}^{2}}+{{\left( \text{BC} \right)}^{2}}\cdots \cdots \cdots \left( 1 \right) $ .
Also $ \Delta ADB $ is right angled triangle with $ \angle D={{90}^{\circ }} $ . So using Pythagoras theorem,
 $ {{\left( \text{BD} \right)}^{2}}={{\left( \text{AD} \right)}^{2}}+{{\left( \text{AB} \right)}^{2}}\cdots \cdots \cdots \left( 2 \right) $ .
Now we know that the opposite sides in a rectangle are equal therefore, AD = BC. Putting it in equation (2) we get, $ {{\left( \text{BD} \right)}^{2}}={{\left( \text{AB} \right)}^{2}}+{{\left( \text{BC} \right)}^{2}}\cdots \cdots \cdots \left( 3 \right) $ .
From (1) and (3) we can see that $ {{\left( \text{AC} \right)}^{2}}={{\left( \text{BD} \right)}^{2}} $ .
Taking square root on both sides we get, AC = BD.
Hence diagonal of a rectangle are equal to each other.
Now for checking if they are perpendicular. Let us assume that $ \angle AOB={{90}^{\circ }}\text{ and }\angle BOC={{90}^{\circ }} $ .
Then in triangle $ \Delta AOB\text{ and }\Delta BOC $ we have, $ \angle AOB\text{ and }\angle BOC $ .
OB = OB
AO = OC (diagonals of a rectangle bisect each other).
So triangles $ \Delta AOB\text{ and }\Delta BOC $ will be congruent by SAS criteria. This implies that AB = BC.
But AB = BC only in the case of square whereas we have a rectangle whose adjacent sides may not be equal. These constraints our assumption.
Therefore, $ \angle AOB $ need to be equal to $ {{90}^{\circ }} $ . Similarly, $ \angle BOC $ need to be equal to $ {{90}^{\circ }} $ .
Hence diagonal of a rectangle need not be perpendicular to each other.
So the given statement is not true.

Note:
Students should note that a square is a type of rectangle that has diagonals that are perpendicular to each other. So the statement is not entirely false but it is just not applicable to all rectangles. Try to keep in mind the properties of the quadrilateral.