
Diagonals of a rhombus are 12cm and 16cm respectively, then find the side of the rhombus and its perimeter.
Answer
531k+ views
Hint: Rhombus is a two dimensional shape with four equal sides and four angles which can or cannot be
Complete step by step answer:
According to given data,
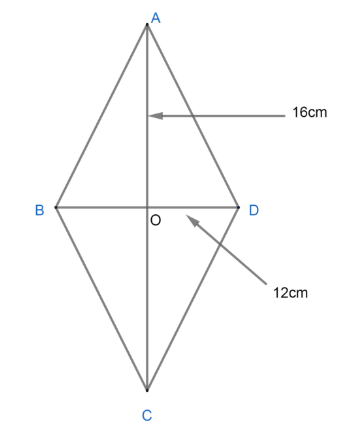
Shape ABCD is a rhombus and O is the intersecting point of both diagonals which is bisecting both diagonals, and both diagonals are perpendicular to each other according to definition.
So,
According to Pythagoras Theorem,
And we know the perimeter of the rhombus is equal to 4a where ‘a’ is the length of a side.
So, Perimeter of rhombus
Hence, the side is 10cm and the perimeter is 40cm.
Note: Here we should have appropriate knowledge of general shapes, some time lack of knowledge can confuse us in easy problems. By the way here we can use direct formula to find out the length of side which is
Complete step by step answer:
According to given data,

Shape ABCD is a rhombus and O is the intersecting point of both diagonals which is bisecting both diagonals, and both diagonals are perpendicular to each other according to definition.
So,
According to Pythagoras Theorem,
And we know the perimeter of the rhombus is equal to 4a where ‘a’ is the length of a side.
So, Perimeter of rhombus
Hence, the side is 10cm and the perimeter is 40cm.
Note: Here we should have appropriate knowledge of general shapes, some time lack of knowledge can confuse us in easy problems. By the way here we can use direct formula to find out the length of side which is
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

How do you prove that the diagonals of a rectangle class 10 maths CBSE




