
What does a dot product equal to one mean?
Answer
404.4k+ views
1 likes
Hint: We need to have a basic idea of what a dot product actually means. There are many definitions of dot products. We use the basic definition which states dot product or scalar product is given by:
Complete step by step solution:
The dot product is also called a scalar product. This states that multiplying any two vectors will give us a single vector which is scalar. Here we have considered the vectors as A and B
Scalar product or dot product is basically defined as a product of 2 vectors along with the angle of cosine between them. Look at the formula above , we have written 2 terms
According to the question they have given that the dot product is 1. It means that
Or,
This is possible only when :
And
Hence the 2 vectors are parallel. To get better idea look at the image shown below:
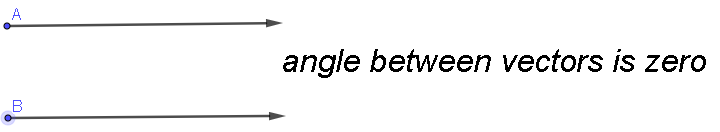
Now the fact that the angle between 2 vectors is 0 means that the vector could be parallel or antiparallel as shown in the image below;

Hence if the dot product is 1 it means that the two given vectors are parallel. And if the dot product is -1 it means that the two given vectors are antiparallel.
Additional Information:
Note:
We should remember that the angle being 0 doesn’t mean that the vectors are only parallel; they can also be antiparallel. Thus when the dot product is 1 it means the vectors are parallel and when the dot product is-1 it means the vectors are anti-parallel.
Complete step by step solution:
The dot product is also called a scalar product. This states that multiplying any two vectors will give us a single vector which is scalar. Here we have considered the vectors as A and B
Scalar product or dot product is basically defined as a product of 2 vectors along with the angle of cosine between them. Look at the formula above , we have written 2 terms
According to the question they have given that the dot product is 1. It means that
Or,
This is possible only when :
And
Hence the 2 vectors are parallel. To get better idea look at the image shown below:
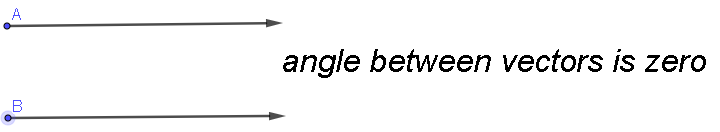
Now the fact that the angle between 2 vectors is 0 means that the vector could be parallel or antiparallel as shown in the image below;

Hence if the dot product is 1 it means that the two given vectors are parallel. And if the dot product is -1 it means that the two given vectors are antiparallel.
Additional Information:
Note:
We should remember that the angle being 0 doesn’t mean that the vectors are only parallel; they can also be antiparallel. Thus when the dot product is 1 it means the vectors are parallel and when the dot product is-1 it means the vectors are anti-parallel.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Class 10 Question and Answer - Your Ultimate Solutions Guide

Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




