
What does sonar equipment measure?
Answer
387.9k+ views
Hint: We measure distance by taking two points (initial and final) and we measure the length span between them. Sometimes this method is not quite applicable so we sent a ray and let it bounce back to yourself then we find the distance by dividing the total length covered by the ray into half
Pythagoras Theorem.
Complete Step By Step Answer:
Sonar also called sound navigation and ranging is a technique that uses sound propagation to navigate, measure distances (ranging), communicate with or detect objects on or under the surface of the water, such as other vessels.
In this process we send a sound ray in water by the transmitter, the ray then bounces back to the receiver.
We then note the time after which the ray has reached us.
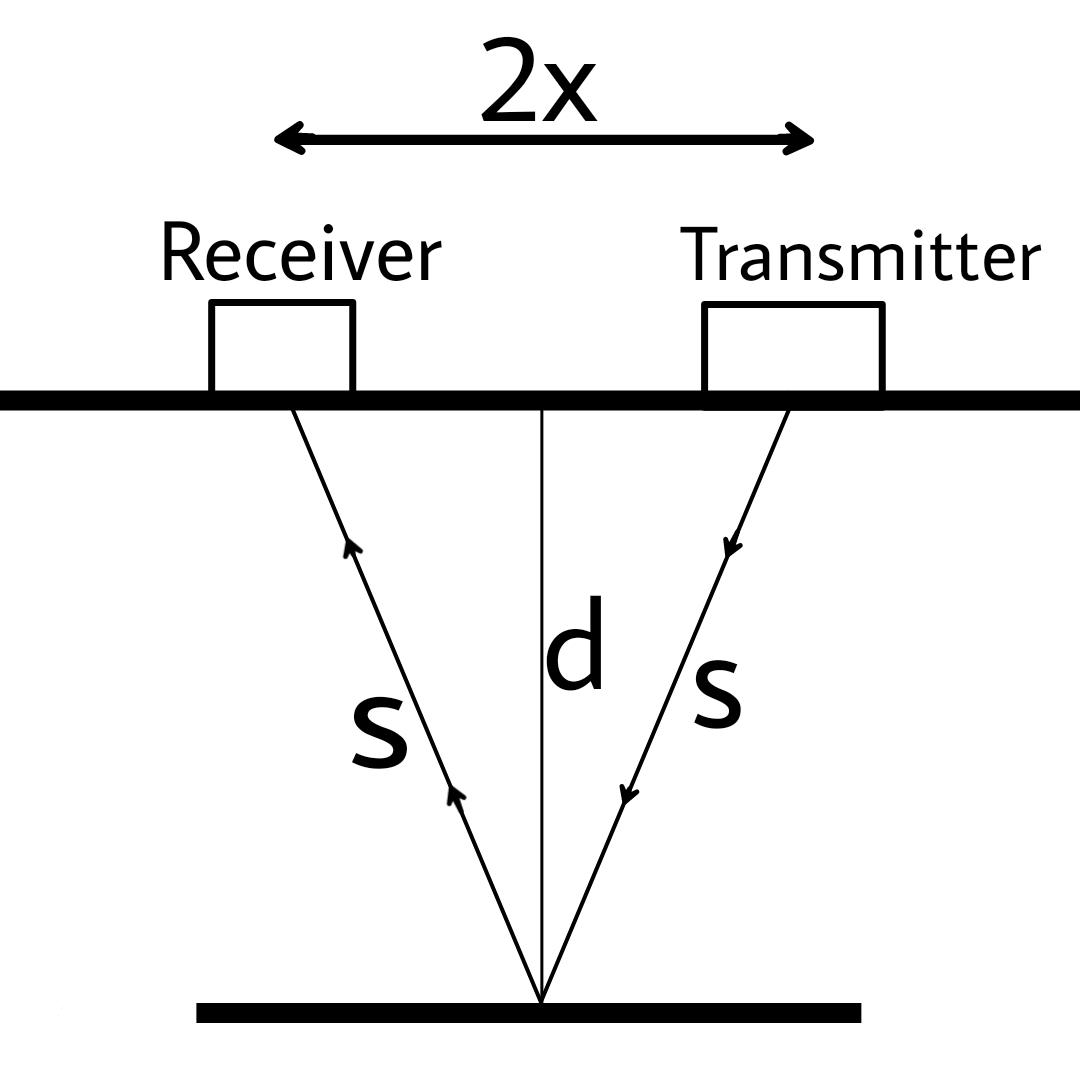
The above figure shows the propagation of the ray inside water. Where
Since, we know the time and the velocity of the sound ray we have the value of (hypotenuse).
Now we use the Pythagoras theorem to find the value of the depth.
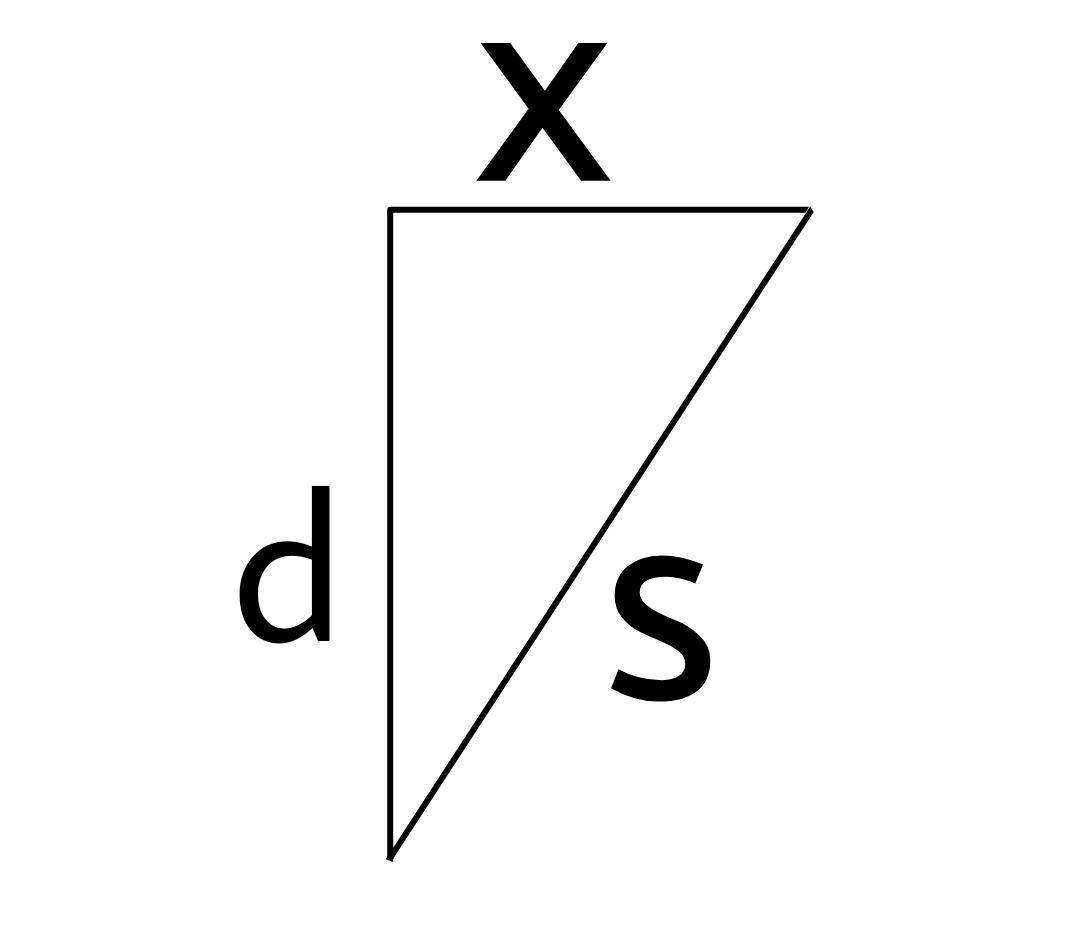
Now,
Thus, with the above formula we can find the depth of the sea.
Note:
Sometimes there can be rocks which can't give us the actual measurement of the depth so we send many rays in a particular large area in order to confirm the actual depth of the sea bed. And also at times If we know that the depth is too high and the distance between the transmitter and the receiver is very low we can neglect this distance as it the depth almost becomes equal to the distance the rays travelled
Pythagoras Theorem.
Complete Step By Step Answer:
Sonar also called sound navigation and ranging is a technique that uses sound propagation to navigate, measure distances (ranging), communicate with or detect objects on or under the surface of the water, such as other vessels.
In this process we send a sound ray in water by the transmitter, the ray then bounces back to the receiver.
We then note the time after which the ray has reached us.

The above figure shows the propagation of the ray inside water. Where
Since, we know the time and the velocity of the sound ray we have the value of (hypotenuse).
Now we use the Pythagoras theorem to find the value of the depth.
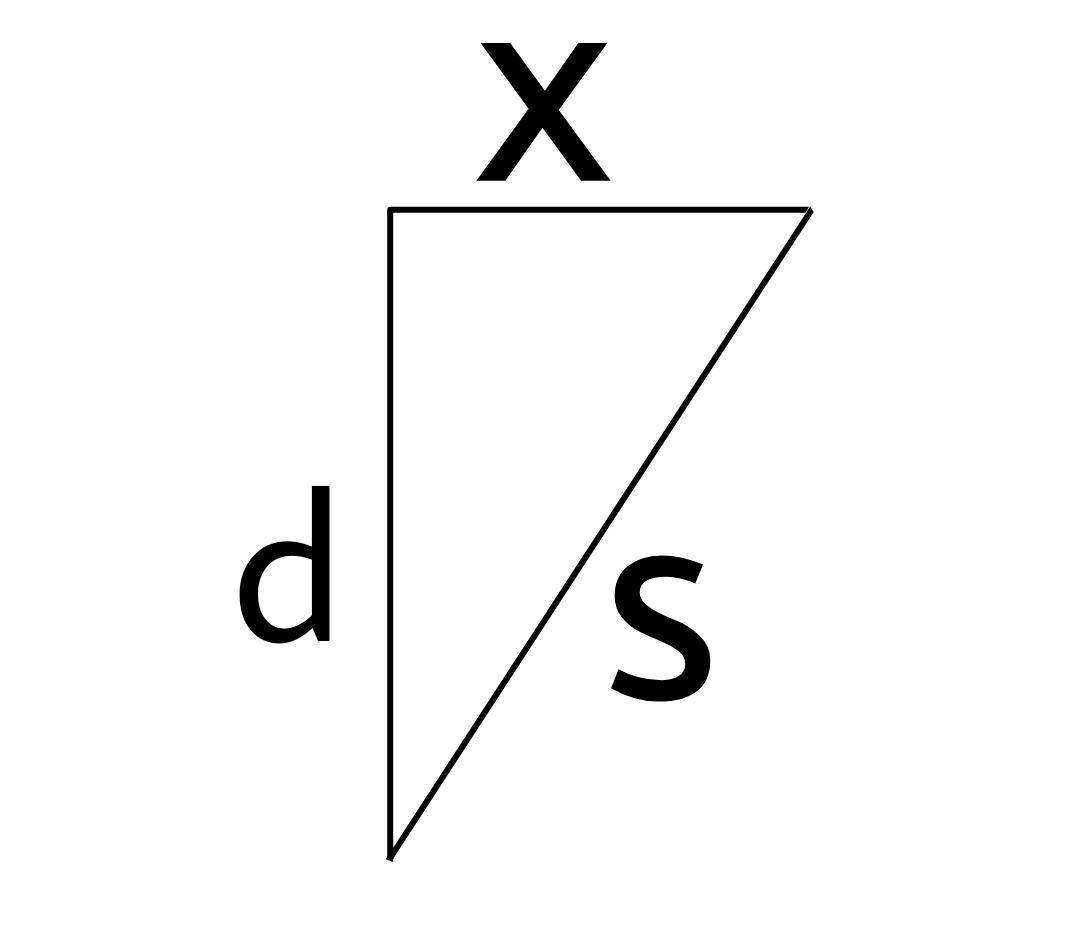
Now,
Thus, with the above formula we can find the depth of the sea.
Note:
Sometimes there can be rocks which can't give us the actual measurement of the depth so we send many rays in a particular large area in order to confirm the actual depth of the sea bed. And also at times If we know that the depth is too high and the distance between the transmitter and the receiver is very low we can neglect this distance as it the depth almost becomes equal to the distance the rays travelled
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
EMI starts from ₹3,487.34 per month
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




