Question

What is the domain and range of $y=\sec x$?

Answer 1

Hint: To solve this question, we must know that domain is the value of $x$ for which a function is defined, while range is the set of all value attained by the function in its domain. The range is decided by creating a graph. So, we will create a graph for the given range and then we will decide the domain by excluding the real numbers at which the function becomes non defined.

Complete step-by-step solution:
In the question, we have been asked to find the domain and the range of $y=\sec x$.
Now, we know that we have function as $y=\sec x$ and using the reciprocal identity of trigonometric functions, we have $\sec x=\dfrac{1}{\cos x}$.
We also know that the function will become not defined when we have $\cos x=0$. We also know that $\cos x=0$ is possible when the value of $x=\left( 2n+1 \right)\dfrac{\pi }{2}$, where $n\in $ natural number.
Now let us create the graph for this as below.

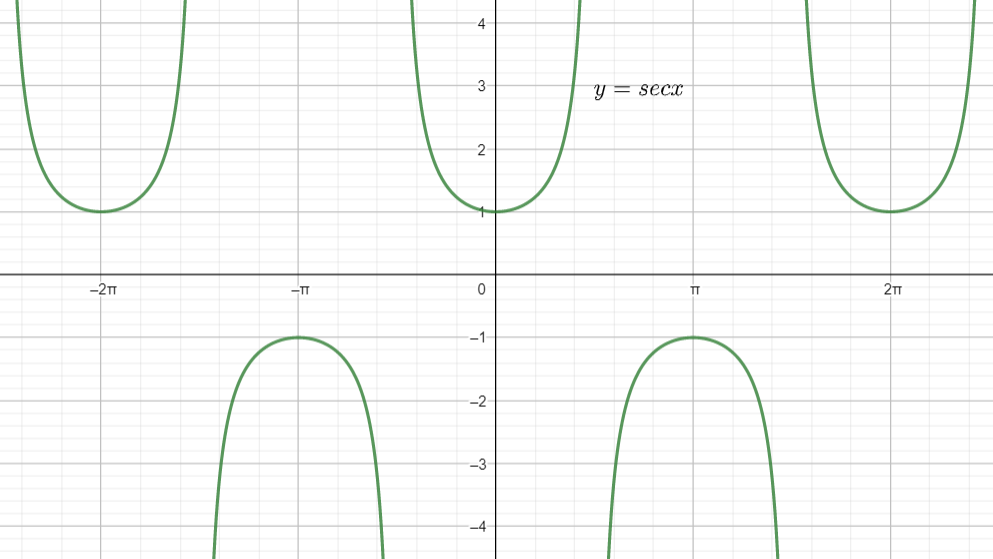
Looking at the graph, we find that the range can be found to be,
$\left( -\infty ,-1 \right]\cup \left[ 1,\infty \right)$ or $R-\left( -1,1 \right)$.

Note: There is another approach to solve the given question. It would be to proceed with finding the derivative, local or global maxima and minima. Some common mistakes that can occur while solving this question include creating a wrong graph which would lead to incorrect values. And we should make sure to check for the proper domain.