Question

Draw a circle with centre at point O and radius 5 cm. Draw its chord AB, draw the perpendicular bisector of line segment AB. Does it pass through the centre of the circle?

Answer 1

Hint: Use the compass and scale to measure the radius and rotate the compass to ${{360}^{\circ }}$ with the measure of the gap as radius of circle, chord is a line segment joining two points lying on a circle and draw perpendicular bisector line and justify whether it passes through centre of circle.

Complete step-by-step answer:
Perpendicular bisector of a line segment can be drawn by joining points of intersection of arcs opposite to the line segment, made by the compass from the extreme points of the line segments with the measure of the gap of the compass be greater than half of the length of line segment. As we need to draw a circle of radius 5 cm. So, we need to take a point on the paper and use the compass and scale measure 5 cm distance. And now draw the circle by taking the point on the paper as the centre of the circle.

Construction Steps:
i) Take a point on the sheet and name it as O.
ii) Put the sharp point of compass to the point O.
iii) Measure the distance of 5 cm by compass using the scale i.e. make the gap of compass as 5cm.
iv) Now, draw the circle by rotating the compass to ${{360}^{\circ }}$.
So, take two points A and B on the circle and join them to get chord in the following way:

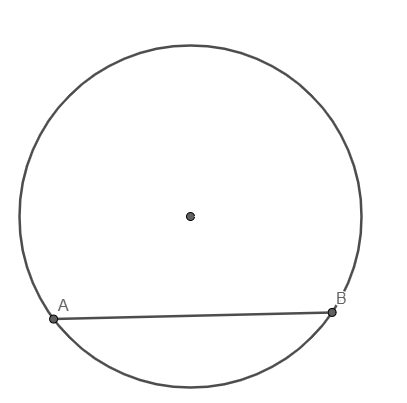
For drawing perpendicular distance, follow the construction steps:

Construction Steps:
i) Put the edge point of the compass at point A and measure more than half distance (should be more than half distance of AB) of AB just by observation and draw arcs to the both sides of the line segment AB.
ii) Similarly, to the above step, put the edge point of the compass at point B, and draw the arcs to both sides with the same distance taken in the very first step.
iii) Name the points of intersection of arcs to opposite side of AB as C and D.
iv) Now join point C and D.
v) Line CD will act as a perpendicular bisector of AB.

From the constructed diagram we can say that the perpendicular bisector of line segment AB passes through the centre of the circle.

Note: Be careful that the gap of the compass for making arcs while drawing a perpendicular bisector should be more than half of the chord (line segment given) in the circle. One can measure the full length of the (line segment) chord for drawing a perpendicular bisector as well, but can’t take the gap less than half of that.
If the perpendicular bisector of the chord is not passing through the centre, then the construction made has gone wrong somewhere. As it is the property of the circle that the line perpendicular to the chord and dividing it in equal part will pass through the centre always.