
Draw a triangle ABC with side BC = 6cm, AB = 5cm and
Answer
479.1k+ views
Hint: In this question, we are given an angle and two sides of a triangle. Firstly, we have to draw a proper triangle using given measurements and then we have to construct another triangle whose sides are
Complete step by step answer:
Let us draw a triangle ABC with sides BC = 6cm, AB = 5cm and
Following are the steps of construction to draw it.
(1): Firstly, let us draw a line segment BC of length 6cm using a ruler.
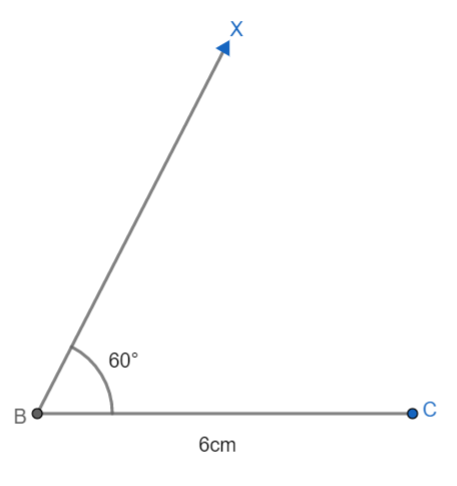
(2): Now with B as center, let us draw an angle of
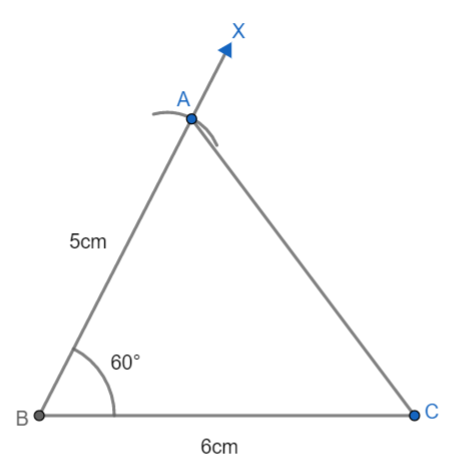
(3): Now with B as center and 5cm as radius on the compass, let us draw arc on the ray BX which cuts the ray BX at point A. Join A to C, we get,
Hence
Now let us make a triangle which is
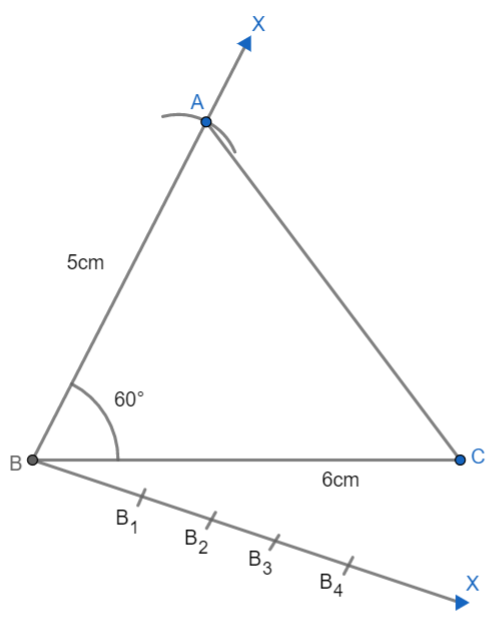
(1): From B, let us draw any ray BX which makes same acute with side BC on the opposite to vertex A. Then let us mark 4 points
(1): Let us join
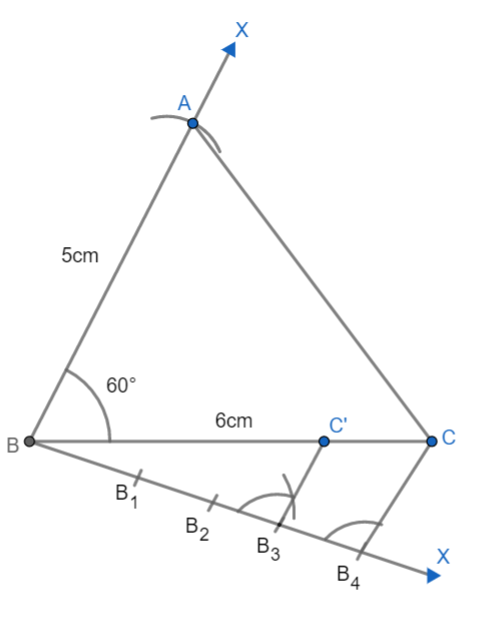
(3): Now similarly, draw a line parallel to AC from C' to the line AB we get:

The triangle A'C'B is our required new triangle.
Note: Students should know how to draw parallel lines, angles using compass and ruler only as they are basics for these constructions. Make sure that, pencil is sharp and the compass is tight. Draw all arcs carefully.
Complete step by step answer:
Let us draw a triangle ABC with sides BC = 6cm, AB = 5cm and
Following are the steps of construction to draw it.
(1): Firstly, let us draw a line segment BC of length 6cm using a ruler.

(2): Now with B as center, let us draw an angle of

(3): Now with B as center and 5cm as radius on the compass, let us draw arc on the ray BX which cuts the ray BX at point A. Join A to C, we get,

Hence
Now let us make a triangle which is
(1): From B, let us draw any ray BX which makes same acute with side BC on the opposite to vertex A. Then let us mark 4 points

(1): Let us join

(3): Now similarly, draw a line parallel to AC from C' to the line AB we get:

The triangle A'C'B is our required new triangle.
Note: Students should know how to draw parallel lines, angles using compass and ruler only as they are basics for these constructions. Make sure that, pencil is sharp and the compass is tight. Draw all arcs carefully.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What are the public facilities provided by the government? Also explain each facility




