
Draw the appropriate Venn diagram for each of the following:
(i)
(ii)
(iii)
(iv)
Answer
487.8k+ views
Hint: You can break the complex Venn diagrams into easier forms then use them to create the main Venn diagram it will help us understand the basics also.
Complete step-by-step answer:
Let’s start with the 1st part that is
So, for drawing Venn diagram of
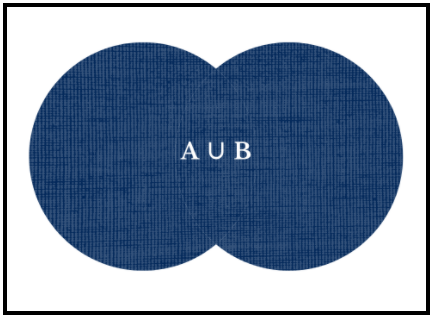
So. For drawing Venn diagram of
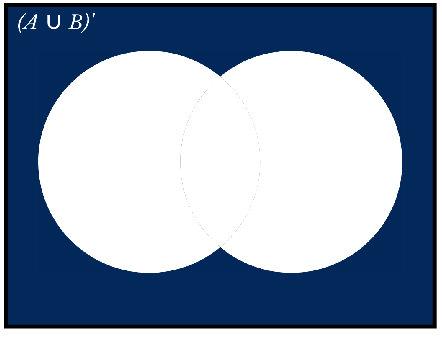
Here
Let’s go on to the 2nd part that is
So, for drawing Venn diagrams of
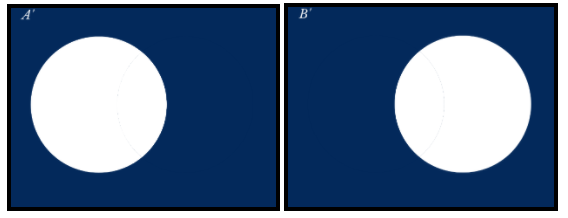
Now, to get a Venn diagram of
After Overlapping Venn Diagrams of
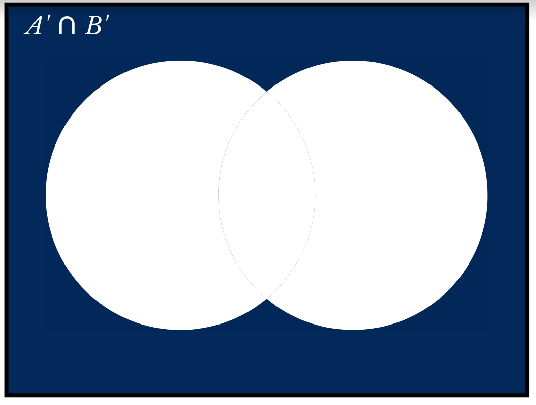
Let’s go on to the 3rd part now, that is
So, for drawing Venn diagram of

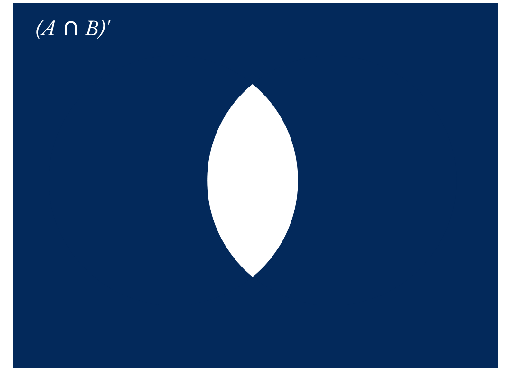
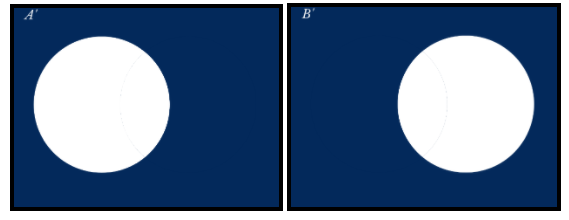
Now, to get the Venn diagram of
After removing it we get this Venn diagram which only consists of
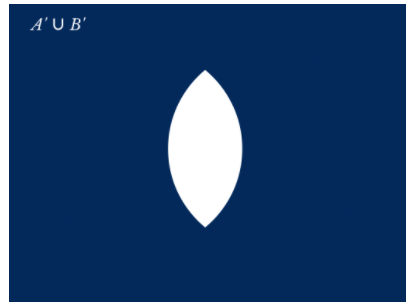
Additional Information: This can be done easily with the use of De Morgan's laws
Note: If we look closely at all four questions, we can easily state that these are the proofs of De Morgan's laws. Using these four we can easily prove both of De Morgarn’s laws.
Complete step-by-step answer:
Let’s start with the 1st part that is
So, for drawing Venn diagram of

So. For drawing Venn diagram of

Here
Let’s go on to the 2nd part that is
So, for drawing Venn diagrams of

Now, to get a Venn diagram of
After Overlapping Venn Diagrams of

Let’s go on to the 3rd part now, that is
So, for drawing Venn diagram of

Now, To get the Venn diagram of

Let’s go on to the 4th part now, that is
So, for drawing a Venn diagram of

Now, to get the Venn diagram of
After removing it we get this Venn diagram which only consists of

Additional Information: This can be done easily with the use of De Morgan's laws
Note: If we look closely at all four questions, we can easily state that these are the proofs of De Morgan's laws. Using these four we can easily prove both of De Morgarn’s laws.
Watch videos on
Draw the appropriate Venn diagram for each of the following:
(i)
(ii)
(iii)
(iv)
(i)
(ii)
(iii)
(iv)

Class 11 MATHS NCERT EXERCISE 1.5 (Question - 5) | Sets Class 11 Chapter 1| NCERT | Ratan Kalra Sir
Subscribe
 Share
Share likes
289 Views
1 year ago
Latest Vedantu courses for you
Grade 8 | CBSE | SCHOOL | English
Vedantu 8 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
EMI starts from ₹3,775 per month
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells



 Watch Video
Watch Video
