
Draw the frequency polygon of the following data using histogram.
Class interval 0-10 10-20 20-30 30-40 40-50 50-60 Frequency 5 10 25 16 12 8
| Class interval | 0-10 | 10-20 | 20-30 | 30-40 | 40-50 | 50-60 |
| Frequency | 5 | 10 | 25 | 16 | 12 | 8 |
Answer
442.2k+ views
Hint: We are first going to represent the above data in the form of a histogram using a bar graph. We have this graph plotted such that the horizontal axis consists of the class interval and the vertical axis contains the frequency. We shall then take the midpoints of these rectangular bars and plot a corresponding graph for the frequency polygon.
Complete step-by-step solution:
Consider the given question with the class intervals ranging from 0 all the way up to 60. This will form the horizontal axis for our bar graph. Now, the vertical axis contains values that are represented by the frequency given in the question. Using this data, we plot the bar graph as shown in the figure below.
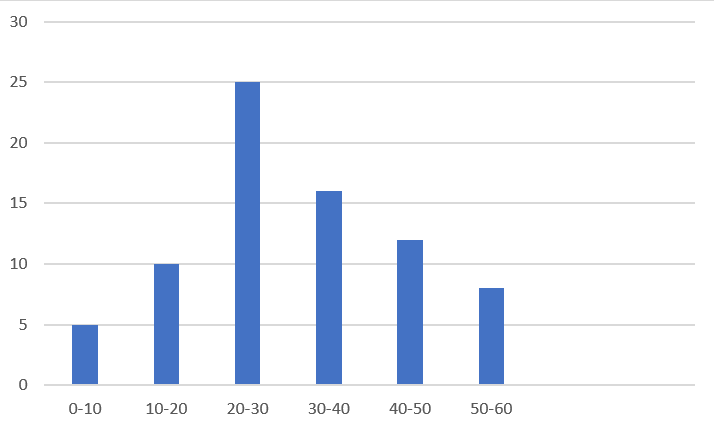
We shall find the mid points of each bar and this can be done by adding the upper limit and lower limit and dividing by 2.
For the first bar having a frequency 5,
Similarly, for the second bar having a frequency 10,
Similarly, for the third bar having a frequency 25,
Similarly, for the fourth bar having a frequency 16,
Similarly, for the fifth bar having a frequency 12,
Similarly, for the sixth bar having a frequency 8,
Now, using this data, we plot a graph with points at these locations and they are connected by straight lines as shown in the figure. This represents the frequency polygon.
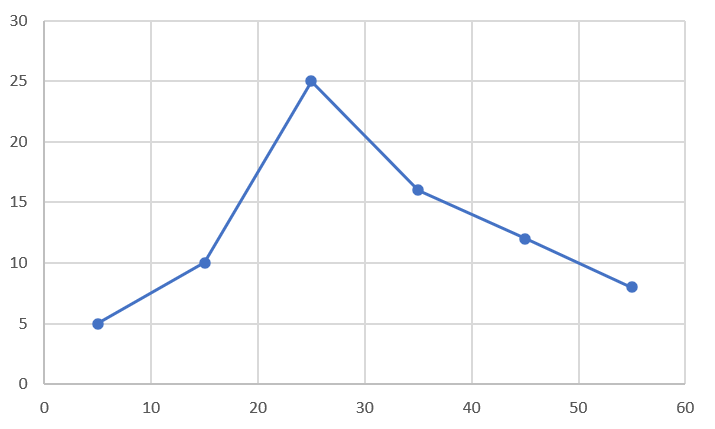
Note: It is to note that we can directly draw the frequency polygon by considering the mid points of each range and plotting for the corresponding frequency values. We have drawn additional histograms here for our better understanding. We are not required to draw the histogram for the given question.
Complete step-by-step solution:
Consider the given question with the class intervals ranging from 0 all the way up to 60. This will form the horizontal axis for our bar graph. Now, the vertical axis contains values that are represented by the frequency given in the question. Using this data, we plot the bar graph as shown in the figure below.

We shall find the mid points of each bar and this can be done by adding the upper limit and lower limit and dividing by 2.
For the first bar having a frequency 5,
Similarly, for the second bar having a frequency 10,
Similarly, for the third bar having a frequency 25,
Similarly, for the fourth bar having a frequency 16,
Similarly, for the fifth bar having a frequency 12,
Similarly, for the sixth bar having a frequency 8,
Now, using this data, we plot a graph with points at these locations and they are connected by straight lines as shown in the figure. This represents the frequency polygon.

Note: It is to note that we can directly draw the frequency polygon by considering the mid points of each range and plotting for the corresponding frequency values. We have drawn additional histograms here for our better understanding. We are not required to draw the histogram for the given question.
Latest Vedantu courses for you
Grade 8 | CBSE | SCHOOL | English
Vedantu 8 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




