
Draw the graph of
Answer
494.1k+ views
Hint: We see that the given equation of curve is in implicit form
Complete step-by-step answer:
We have the given equation of the curve in the question as
Here the condition is the non-negative constraints
We can see that above function is clearly not defined for

We plot the obtained points
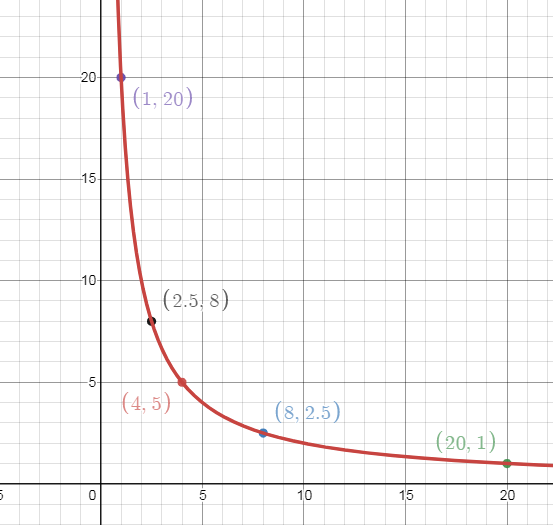
We are also asked to use the graph to find
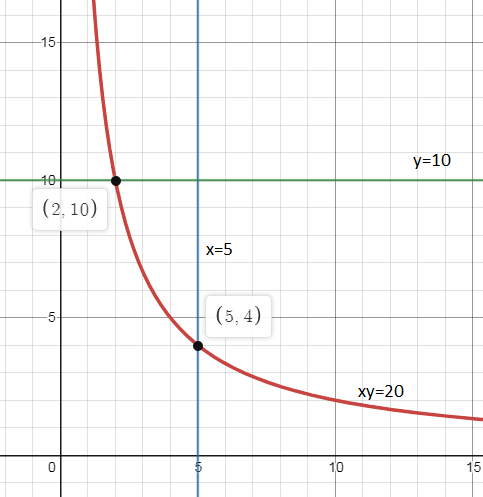
We observe that the line
Note: We can verify the points of intersection by putting them in the equation of the curve. The plot we obtained is the plot of a rectangular hyperbola whose standard equation is given by
Complete step-by-step answer:
We have the given equation of the curve in the question as
Here the condition is the non-negative constraints
We can see that above function is clearly not defined for

We plot the obtained points

We are also asked to use the graph to find

We observe that the line
Note: We can verify the points of intersection by putting them in the equation of the curve. The plot we obtained is the plot of a rectangular hyperbola whose standard equation is given by
Recently Updated Pages
Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Class 10 Question and Answer - Your Ultimate Solutions Guide

Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE

What are the public facilities provided by the government? Also explain each facility




