
Draw the shape of five d-orbitals.
Answer
483k+ views
Hint:. Atomic orbitals:- The three-dimensional space around the atom of an element in which the electron density is maximum. It means the probability of finding an electron is maximum.
Complete step by step answer:
The transition metal ions of the outermost d-orbitals are not completely filled with electrons and hence, they can easily take and give electrons.
For d-orbitals the value of l =
As we see that there are five magnetic quantum numbers, so, the d-orbital will have five orientations. It means, five types of shapes of d-orbital in three-dimensional space.
So, depending upon the axes along which or between which the electron clouds are obtained, different names and shapes are given as;
It is clear from the above names of the five d-orbitals that in which position will be assigned to them in the xyz coordinates, so the shapes are:
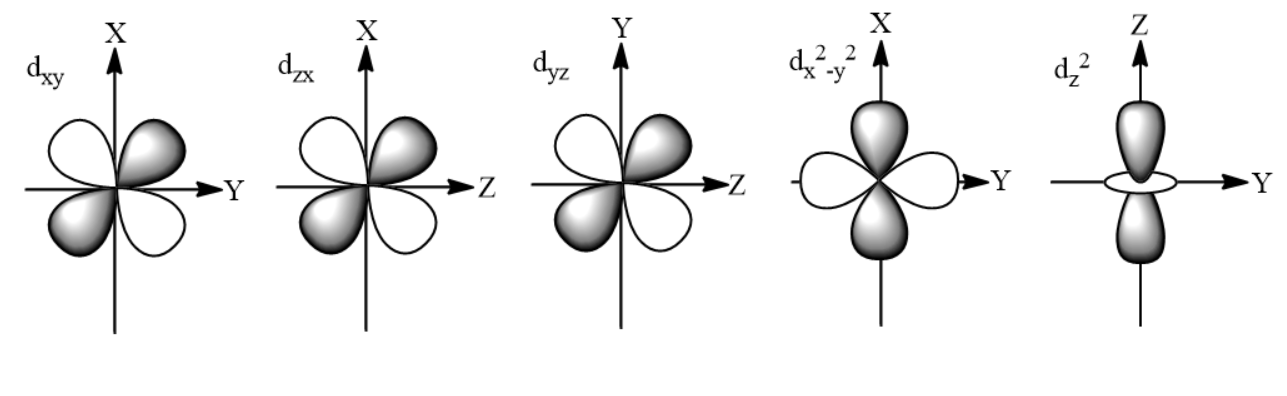
Additional Information:
Significance of four quantum numbers:
The principal quantum number
The azimuthal quantum number
The magnetic quantum number
The spin quantum number
Note: The possibility to make a mistake is that
Complete step by step answer:
The transition metal ions of the outermost d-orbitals are not completely filled with electrons and hence, they can easily take and give electrons.
For d-orbitals the value of l =
As we see that there are five magnetic quantum numbers, so, the d-orbital will have five orientations. It means, five types of shapes of d-orbital in three-dimensional space.
So, depending upon the axes along which or between which the electron clouds are obtained, different names and shapes are given as;
It is clear from the above names of the five d-orbitals that in which position will be assigned to them in the xyz coordinates, so the shapes are:

Additional Information:
Significance of four quantum numbers:
The principal quantum number
The azimuthal quantum number
The magnetic quantum number
The spin quantum number
Note: The possibility to make a mistake is that
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Why is steel more elastic than rubber class 11 physics CBSE

Explain why a There is no atmosphere on the moon b class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE




