
What is the eccentricity of a hyperbola?
Answer
449.4k+ views
Hint: Eccentricity of a hyperbola is defined as
Complete step by step solution:
We know that hyperbola is a collection of a set of points, whose difference of distances from two fixed points is a positive constant. These fixed points are called foci, and are shown as A and A’ in the figure below. Here, the origin is the centre of the hyperbola, and the intersecting points of the line joining the two foci and the conic section are called vertices of the hyperbola.
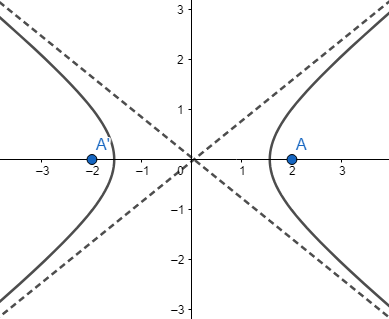
We are well aware that hyperbola is a conic section, and has two branches.
Hyperbola can also be defined in terms of eccentricity, but for that, we first need to understand what eccentricity means.
Eccentricity is a measure of the deviation occurred in the circularity of any conic section.
So mathematically, we can define eccentricity of any conic section as
Where e is the eccentricity of the conic section,
c is the distance between centre and the focus, and
a is the distance between centre and the vertex.
Now, we can define hyperbola in terms of eccentricity as the conic section whose eccentricity is greater than 1, that is, e > 1.
We know that the general equation of a hyperbola is
where, a is the length of semi major axis,
and b is the length of semi minor axis.
So, in other words we can define hyperbola as a conic section in which the distance from a fixed point is greater than the distance from a fixed line, called the directrix.
Hence, for a hyperbola, eccentricity e > 1.
We know that eccentricity for a hyperbola is expressed as
Note: We should not consider the a in eccentricity
We must also note that the above general equation of hyperbola is valid when the centre of the hyperbola is the origin (0,0).
Complete step by step solution:
We know that hyperbola is a collection of a set of points, whose difference of distances from two fixed points is a positive constant. These fixed points are called foci, and are shown as A and A’ in the figure below. Here, the origin is the centre of the hyperbola, and the intersecting points of the line joining the two foci and the conic section are called vertices of the hyperbola.

We are well aware that hyperbola is a conic section, and has two branches.
Hyperbola can also be defined in terms of eccentricity, but for that, we first need to understand what eccentricity means.
Eccentricity is a measure of the deviation occurred in the circularity of any conic section.
So mathematically, we can define eccentricity of any conic section as
Where e is the eccentricity of the conic section,
c is the distance between centre and the focus, and
a is the distance between centre and the vertex.
Now, we can define hyperbola in terms of eccentricity as the conic section whose eccentricity is greater than 1, that is, e > 1.
We know that the general equation of a hyperbola is
where, a is the length of semi major axis,
and b is the length of semi minor axis.
So, in other words we can define hyperbola as a conic section in which the distance from a fixed point is greater than the distance from a fixed line, called the directrix.
Hence, for a hyperbola, eccentricity e > 1.
We know that eccentricity for a hyperbola is expressed as
Note: We should not consider the a in eccentricity
We must also note that the above general equation of hyperbola is valid when the centre of the hyperbola is the origin (0,0).
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

What is the difference between superposition and e class 11 physics CBSE

State and prove Bernoullis theorem class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light




