
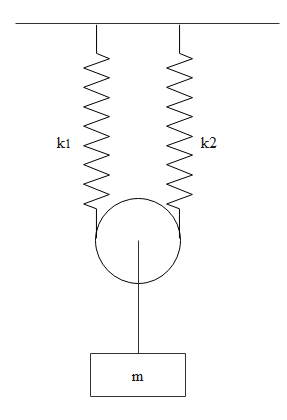
What is the elongation in the two springs.

Answer
494.4k+ views
Hint: This problem can be solved just like we solve the pulley string problem. The weight of the block will transfer to the string attached to it and then it will transfer to the two springs attached to it. Tension produced in each spring will be equal to its spring force.
Formula used:
Complete answer:
When the block is attached to the pulley, due to its weight tension is created in the string which is attached to the pulley.
Now this pulley is attached to the two springs. Here our assumptions are pulley is massless and string is massless and springs are also massless. There is no friction between the pulley and the string or springs.
If there is friction and pulleys have a mass then torque will be acted on pulleys due to friction and then pulley will rotate.
The weight of the block is
This weight will be the tension in the string which is attached to the pulley.
Now this tension will be divided among the springs.
Since there will be a string which will be connecting two springs and holds the pulley in position, the two springs can be assumed to be connected through that string.
So tension in both springs will be the same in both the springs.
Let us assume the tension in the springs is ‘T’
So the spring force in the spring is nothing but the tension in the spring. So the formula for the tension will be
Where
T is the tension in the spring
K is the spring constant of the spring
‘x’ is the elongation produced in the spring due to the tension.
So
Hence we have got the elongation produced in first spring as
Note:
The same procedure can be followed to find out the effective spring constant of a system so that we can find out the time period of oscillations if the small displacement is given to the block.
Formula used:
Complete answer:
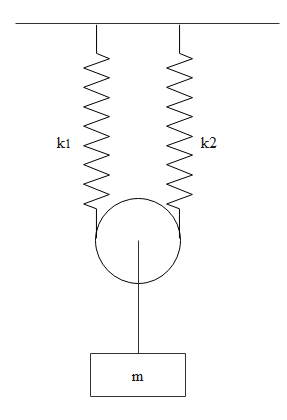
When the block is attached to the pulley, due to its weight tension is created in the string which is attached to the pulley.
Now this pulley is attached to the two springs. Here our assumptions are pulley is massless and string is massless and springs are also massless. There is no friction between the pulley and the string or springs.
If there is friction and pulleys have a mass then torque will be acted on pulleys due to friction and then pulley will rotate.
The weight of the block is
This weight will be the tension in the string which is attached to the pulley.
Now this tension will be divided among the springs.
Since there will be a string which will be connecting two springs and holds the pulley in position, the two springs can be assumed to be connected through that string.
So tension in both springs will be the same in both the springs.

Let us assume the tension in the springs is ‘T’
So the spring force in the spring is nothing but the tension in the spring. So the formula for the tension will be
Where
T is the tension in the spring
K is the spring constant of the spring
‘x’ is the elongation produced in the spring due to the tension.
So
Hence we have got the elongation produced in first spring as
Note:
The same procedure can be followed to find out the effective spring constant of a system so that we can find out the time period of oscillations if the small displacement is given to the block.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




