
What is the emf of the cell C in the circuit shown in figure, if the deflection in the galvanometer is zero, the resistance of the wire is
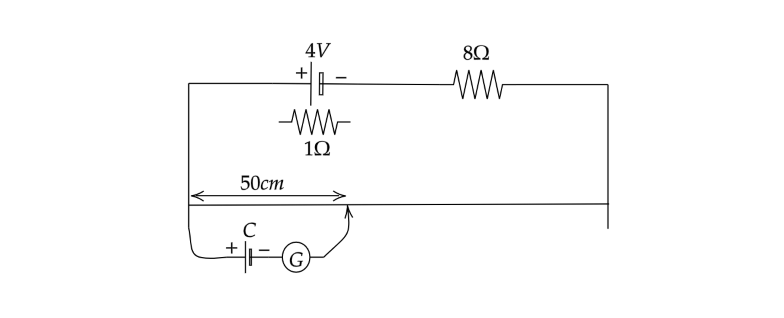

Answer
383.1k+ views
Hint: Here we have to use the meter bridge principle to solve this problem. First we use Kirchhoff voltage law to find the current flowing through the meter bridge circuit. Now zero deflection of the galvanometer shows that voltage across the cell C is equal to the voltage across the
Complete step by step answer:
As per the given problem we have the defection in the galvanometer is zero, the resistance of the wire is
Let I is the amount of current flowing in the circuit.
And r is the internal resistance of the
Now taking a loop across the meter bridge we will get,
Using KVL in the loop ABCD, we have,
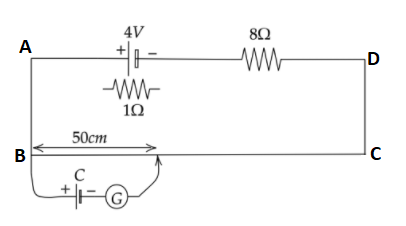
Now putting internal resistance we will get,
Or,
As the galvanometer shows zero deflection we can write,
Voltage across
We know voltage is the product of current and resistance.
As we know,
Now we can write,
And also,
Now putting the values in above equation we will get,
Now on cancelling and on further solving we will get,
Therefore the correct option is (B).
Note:
Remember that the meter bridge is based on the principle of Wheatstone bridge circuit and which states if any point or length in the ratio of two resistance is equal to the ratio of another two resistance then there shall be zero flow of current and the galvanometer connected in it shows zero deflection.
Complete step by step answer:
As per the given problem we have the defection in the galvanometer is zero, the resistance of the wire is
Let I is the amount of current flowing in the circuit.
And r is the internal resistance of the
Now taking a loop across the meter bridge we will get,
Using KVL in the loop ABCD, we have,

Now putting internal resistance we will get,
Or,
As the galvanometer shows zero deflection we can write,
Voltage across
We know voltage is the product of current and resistance.
As we know,
Now we can write,
And also,
Now putting the values in above equation we will get,
Now on cancelling and on further solving we will get,
Therefore the correct option is (B).
Note:
Remember that the meter bridge is based on the principle of Wheatstone bridge circuit and which states if any point or length in the ratio of two resistance is equal to the ratio of another two resistance then there shall be zero flow of current and the galvanometer connected in it shows zero deflection.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




