
Equal volume of two immiscible liquids of densities

Answer
496.8k+ views
1 likes
Hint: Use Bernoulli’s theorem to find the two velocities of efflux. The Bernoulli’s theorem for two points A and B is given as
Formula used:
Complete step-by-step solution:
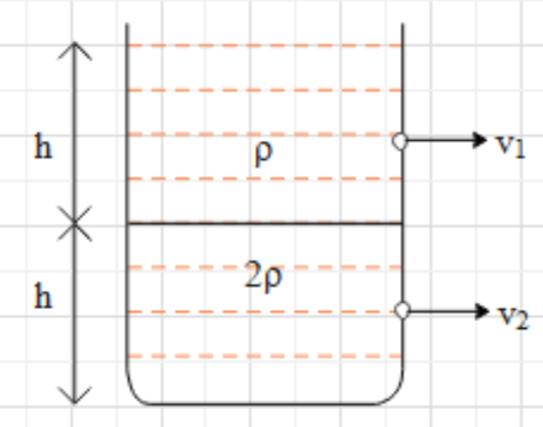
In the given case, there is a container in which two immiscible liquids are present. Since the two liquids are immiscible, they will not interact and mix with each other. Hence, the liquid, which has the least density will be at the top and the liquid with higher density will set down. It is given that the heights of both the liquid layers are equal to h.

Now, we punch two holes in the container. One hole at a depth of
Therefore, there is a flow of both the liquids. When there is a flow of liquid, Bernoulli's theorem comes into role.
Let us apply Bernoulli's theorem, at the points A and B.
Hence, we get,
Therefore, the speed of the liquid coming out from point A is
Now, apply the Bernoulli’s theorem at points B and C.
Therefore, the speed of the liquid coming out from point C is
Therefore,
Hence, the correct option is A.
Note: When a liquid comes out of holes made in the container, the velocity of the liquid is called the velocity of efflux and is given as
Where H is the depth of the hole from the top surface where the liquid is in contact with air.
Therefore,
Then we can find
Formula used:
Complete step-by-step solution:
In the given case, there is a container in which two immiscible liquids are present. Since the two liquids are immiscible, they will not interact and mix with each other. Hence, the liquid, which has the least density will be at the top and the liquid with higher density will set down. It is given that the heights of both the liquid layers are equal to h.

Now, we punch two holes in the container. One hole at a depth of
Therefore, there is a flow of both the liquids. When there is a flow of liquid, Bernoulli's theorem comes into role.
Let us apply Bernoulli's theorem, at the points A and B.
Hence, we get,
Therefore, the speed of the liquid coming out from point A is
Now, apply the Bernoulli’s theorem at points B and C.
Therefore, the speed of the liquid coming out from point C is
Therefore,
Hence, the correct option is A.
Note: When a liquid comes out of holes made in the container, the velocity of the liquid is called the velocity of efflux and is given as
Where H is the depth of the hole from the top surface where the liquid is in contact with air.
Therefore,
Then we can find
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




