Question

Equation of a circle which touch both axis and also the line $x=k\left( k>0 \right)$ is:
A. ${{x}^{2}}+{{y}^{2}}-kx\pm ky+\dfrac{{{k}^{2}}}{4}=0$
B. ${{x}^{2}}+{{y}^{2}}+kx\pm ky+\dfrac{{{k}^{2}}}{4}=0$
C. ${{x}^{2}}+{{y}^{2}}\pm kx+ky+\dfrac{{{k}^{2}}}{4}=0$
D. ${{x}^{2}}+{{y}^{2}}\pm kx-ky+\dfrac{{{k}^{2}}}{4}=0$

Answer 1

Hint: First we need to assume that the distance in the y-axis of the centre of the circle is k and we need to draw the figure of the circle which touches both axis and the line $x=k\left( k>0 \right)$. Then by using the figure we can clearly see the distance marked. And now by this we will find the centre and the radius by this.

Complete step by step answer:
According to the question it is asked to find the equation of the circle which touches both the axis and also this line $x=k\left( k>0 \right)$. Then by using the distance formula between two points with coordinates $\left( {{x}_{1}},{{y}_{1}} \right)$ and $\left( {{x}_{2}},{{y}_{2}} \right)$ as $\sqrt{{{\left( {{x}_{2}}-{{x}_{1}} \right)}^{2}}+{{\left( {{y}_{2}}-{{y}_{1}} \right)}^{2}}}$, we get the value of radius of the circle. Now if we draw the figure for this, then we will consider the centre as $\left( \dfrac{k}{2},\dfrac{k}{2} \right)$ and another as $\left( \dfrac{k}{2},\dfrac{-k}{2} \right)$. Generally we can say that the centre is $\left( \dfrac{k}{2},\pm \dfrac{k}{2} \right)$ and from the centre we can also say that the radius of both the circles is $\dfrac{k}{2}$. So, the figure is as given below:

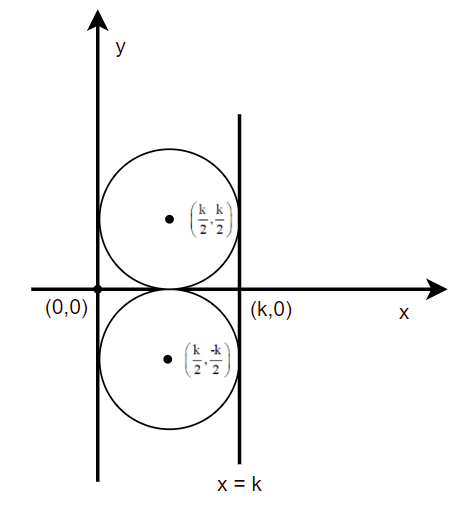
By this we get,
$\begin{align}
  & {{\left( x-\dfrac{k}{2} \right)}^{2}}+{{\left( y\pm \dfrac{k}{2} \right)}^{2}}=\dfrac{{{k}^{2}}}{4} \\
 & \Rightarrow {{x}^{2}}+{{y}^{2}}-kx\pm ky+\dfrac{{{k}^{2}}}{4}=0 \\
\end{align}$
So, the correct answer is “Option A”.

Note: Now, to solve these types of questions we need to know some of the basic formulas to calculate the distance between two points also. So, we use: Let AB be the line with coordinates as $A\left( {{x}_{1}},{{y}_{1}} \right)$ and $B\left( {{x}_{2}},{{y}_{2}} \right)$. Then the distance between the points AB is $\overline{AB}=\sqrt{{{\left( {{x}_{2}}-{{x}_{1}} \right)}^{2}}+{{\left( {{y}_{2}}-{{y}_{1}} \right)}^{2}}}$.