
Explain the formation of
Answer
428.9k+ views
3 likes
Hint: Draw molecular orbital diagram for oxygen molecule. Then, count the number of electrons in bonding molecular orbital and antibonding molecular orbital. After that count the bond order by using the following formula.
Bond Order =
The bond order will tell us the number of bonds that will be formed between two atoms of oxygen molecules.
Complete step by step solution:
The molecular orbital theory is a widely accepted theory for describing the electronic structure of molecules.
The formation of oxygen molecule can be given as –
We know that Oxygen has atomic number = 8. Thus, the electronic configuration for an atom of oxygen in the ground state can be given as –
One atom of oxygen has 8 electrons. Thus, two atoms will possess 16 electrons i.e. Oxygen molecules will have 16 electrons.
The molecular orbital diagram of an Oxygen molecule is as –
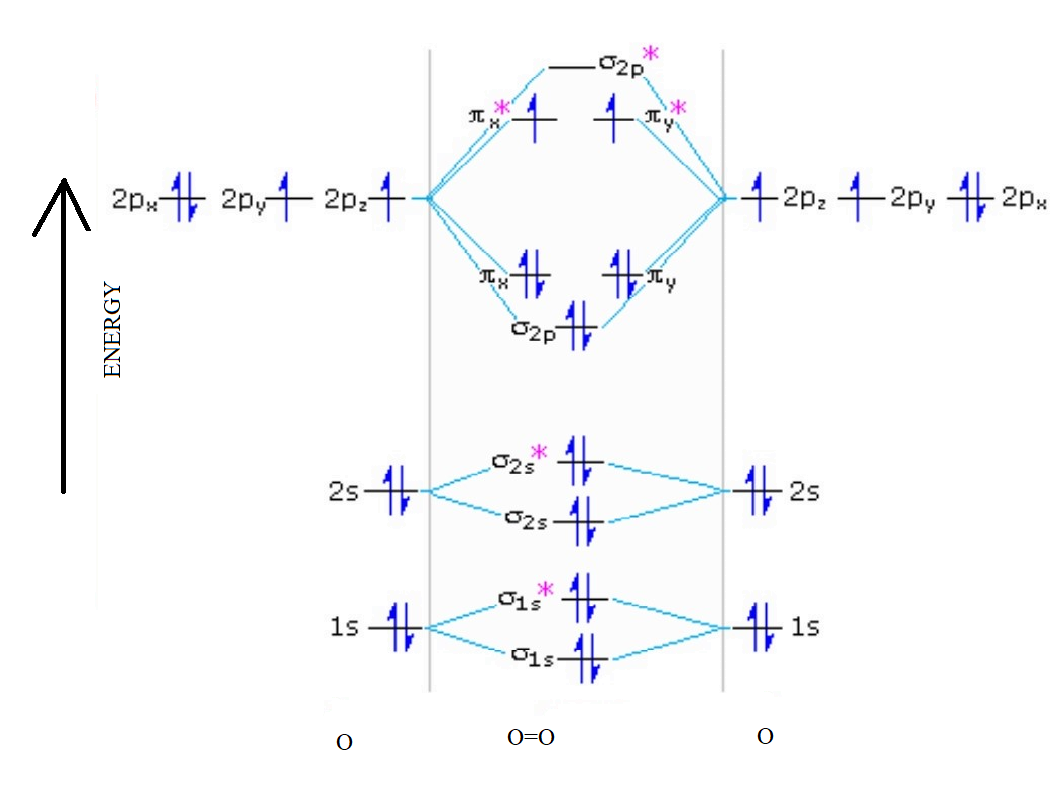
From the diagram, the electronic configuration of oxygen molecule can be written as -
Now, we can calculate the bond order.
We know the formula for bond order is:-
Bond Order =
Thus, Bond Order =
Bond Order =2
Thus, the oxygen molecule has two bonds. i.e., one is
The two electrons in
Note: There are two types of molecular orbital diagrams. The rest of the diagram is the same. The change occurs in two at the point of the splitting of p- orbitals. This should be taken care of so that we choose the right splitting.
Bond Order =
The bond order will tell us the number of bonds that will be formed between two atoms of oxygen molecules.
Complete step by step solution:
The molecular orbital theory is a widely accepted theory for describing the electronic structure of molecules.
The formation of oxygen molecule can be given as –
We know that Oxygen has atomic number = 8. Thus, the electronic configuration for an atom of oxygen in the ground state can be given as –
One atom of oxygen has 8 electrons. Thus, two atoms will possess 16 electrons i.e. Oxygen molecules will have 16 electrons.
The molecular orbital diagram of an Oxygen molecule is as –

From the diagram, the electronic configuration of oxygen molecule can be written as -
Now, we can calculate the bond order.
We know the formula for bond order is:-
Bond Order =
Thus, Bond Order =
Bond Order =2
Thus, the oxygen molecule has two bonds. i.e., one is
The two electrons in
Note: There are two types of molecular orbital diagrams. The rest of the diagram is the same. The change occurs in two at the point of the splitting of p- orbitals. This should be taken care of so that we choose the right splitting.
Latest Vedantu courses for you
Grade 8 | CBSE | SCHOOL | English
Vedantu 8 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




